Collection of Solved Problems in Physics

Change in the Metal Plate's Size during Heating

Task number: 2107

• Notation

  d0 = 36.0 mm = 3.60·10−2 m	diameter of the round hole before heating
  l0	circumference of the round hole before heating
  db = 36.1 mm = 3.61·10−2 m	diameter of the ball
  lb	circumference of the smallest hole through which the ball could pass

  From the Tables:

  α = 2.4·10−5 K−1

  coefficient of linear thermal expansion of aluminium

• Analysis

  The circumference of the hole in the plate is smaller than the circumference of the ball’s projection into the plate’s plane (hereinafter the circumference of the ball). Due to thermal expansion, the dimensions of the plate and the hole in the middle will get larger, if we heat the plate up. The ball will then be able to pass through the hole.

  To solve the task, we have to know the relation for the change in object length with regard to linear thermal expansion. We use this relation to calculate changes in lengths of objects whose one dimension is considerably larger than the other two (e.g. rods or wires). We can imagine that the metal consists of lots of thin metal strips. Then we can use the relation for linear thermal expansion to calculate its enlargement cased by heating.

  Fig. 1: The circumference of the ball is represented by a dashed line around the hole in the plate.

  

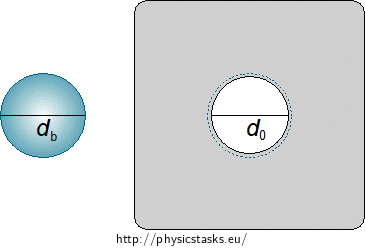
  The circumference of the hole must be enlarged at least to the ball’s circumference. So we write the relation for the enlarged circumference of the hole after heating and substitute the circumference of the ball for it. Therefrom, we express the required temperature change.

• Hint

  Imagine the plate being “cut” into thin concentric rings whose centres lie in the centre of the hole. Express the change in the circumference of such a ring after increasing its temperature by Δt.

• Hint Solition

  If the ring’s initial circumference is l₀ and its temperature is increased by Δt, the final circumference after expansion is l. Expansion will by proportional to the initial length and temperature change. We can express it by the following relation:

  \[Δl = l - l_{\mathrm{0}} = l_{\mathrm{0}}αΔt,\]

  where α is the coefficient of linear thermal expansion of the material of which the ring is made.

  Then we calculate the circumference of the ring after heating from this relation:

  \[l = l_{\mathrm{0}}(1+αΔt).\]

• Solution

  

  We calculate the circumference of the round hole of the diameter d₀ before the heating from:

  \[l_{\mathrm{0}} = πd_{\mathrm{0}}.\tag{1}\]

  If the temperature of the aluminium plate is increased by Δt, the round hole will enlarge and we can apply the following relation for its circumference l:

  \[l = l_{\mathrm{0}}(1+αΔt).\tag{2}\]

  The ball’s diameter is d_b. Therefore, the diameter of the hole through which the ball would pass must be at least equal to d_b. The circumference of such a hole l_b can be calculated from:

  \[l_{\mathrm{b}} = πd_{\mathrm{b}}.\tag{3}\]

  In order for the ball to pass through the hole, its circumference after heating must be at least equal to l_b. So we substitute l for the circumference of the ball l_b in relation (2):

  \[l_{\mathrm{b}} = l_{\mathrm{0}}(1+αΔt).\]

  Now we substitute for relation (1) and (3) in relation (2):

  \[πd_{\mathrm{b}} = πd_{\mathrm{0}}(1+αΔt),\]

  Then we adjust the relation and express Δt from it:

  \[\frac{d_{\mathrm{b}}}{d_{\mathrm{0}}}−1 = αΔt\] \[Δt = \frac{d_{\mathrm{b}}−d_{\mathrm{0}}}{α·d_{\mathrm{0}}}.\tag{4}\]

  Then we substitute for the values from the task assignment in relation (4) and calculate, by how many degrees we have to heat the plate up:

  \[Δt = \frac{3.61·10^{−2}−3.60·10^{−2}}{2.4·10^{−5}·3.60·10^{−2}} °\mathrm{C}\] \[Δt \dot{=} 115.7 °\mathrm{C}.\]

• Answer

  If we want the ball to pass through the round hole, it is necessary to heat the aluminium plate up by at least 115.7 °C.