Change in Internal Energy of an Ideal Gas
Task number: 2176
After undergoing an isochoric process, pressure of 10 l of an ideal gas increased four times. Thereafter, the gas has been cooled isobarically which caused the decrease in volume to one half. Initial pressure of the gas is 100 kPa.
The gas is considered to be a monatomic ideal gas, whose Poisson constant is
\[\kappa = \frac{5}{3}.\]Determine the work of external forces, total heat and change of internal energy of the gas during the described process.
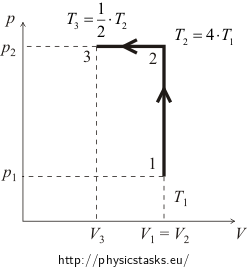
Figure
Hint
Think how we can determine work using the pV diagram.
Consider the temperature changes of an ideal gas during an isochoric increase of pressure and isobaric compression.
Analysis
Total work performed by the external forces is equal to the work done during the isobaric process because no work is performed during the isochoric process. Since the pressure in the isobaric process is constant, the work is equal to the product of the pressure and volume difference.
To calculate the total heat, let us have a look at its change during each process.
In the isochoric process, pressure of the gas is increasing and considering the ideal gas law, so is its temperature. Since neither the gas itself nor the external forces perform any work, its internal energy must have been increased because of supplied heat. This heat can be calculated using the molar heat capacity at constant volume. Its value is determined by the Poisson constant and Meyer's relation.
During the isobaric cooling, temperature of the gas, and thus its internal energy, is decreasing. At the same time the gas is being compressed, which means that the surroundings are performing work. It implies that the gas has to transfer heat to the surroundings. In the calculation, we would also use the fact that, according to the ideal gas law, since the volume of the gas has decreased to one half during the isobaric compression, its temperature has been lowered to one half as well.
Then, the resultant heat is the difference between the heat supplied by the gas and received by it.
Now, just the change in the internal energy of the gas during the process is left.
In case of the isochoric process, performed work is zero, so according to the 1st law of thermodynamics, the change in internal energy is equal to the received or supplied heat. Calculation of this heat has been mentioned above. Internal energy change during the isobaric process can again be calculated using the temperature difference and the molar heat capacity at constant volume (change of internal energy does not depend on the type of the process but only on the temperature change).
Notation
p1 = 100 kPa = 105 Pa initial pressure of the gas V1 = 10 l = 10 dm3 = 0.01 m3 initial volume of the gas \(\kappa = \frac{5}{3}\) Poisson constant W = ? work of the external forces Q = ? total heat ΔU = ? change in the internal energy of the gas during the process Solution: Calculating the Work
First, we determine the work W of the external forces throughout the process. After we realize that no work can be done during the isochoric process, we just need to calculate the work performed in the isobaric process at pressure p2
\[p_{2}=4p_{1}.\]The following formula applies to the work Wp done by the gas (since we are considering the process at constant pressure, we can avoid integration)
\[W_p=p\mathrm{\Delta} V.\]We substitute given values of pressure and volume
\[W_p=p_{2}\left( V_{3}-V_{2}\right)=p_{2}\left(\frac{1}{2} V_{1}- V_{1}\right)=-\frac{1}{2} p_{2}V_{1}.\]Using the relation between p1 and p2, we obtain the final formula for the total work.
\[W_p = - 2p_{1}V_{1},\]where p1 is the initial pressure and V1 is the initial volume.
Negative sign means that the work was not performed by the gas, but the external forces, which corresponds with the isobaric compression. Thus, external forces perform a work
\[W = 2p_{1}V_{1}.\]Solution: Calculating the Heat
When calculating the heat, we need to keep in mind that during the isochoric process, in which pressure increases four times, no work performed and temperature increases to the value T2 = 4T1 (which follows from the fact that according to the ideal gas law, in case of an isochoric process V = const, ratio \(\frac{p}{T}\) is constant as well).
As a result, internal energy of the gas is increasing, so the heat must be supplied to the system. For the amount of the heat received by the system Q1 (and increase of the internal energy ΔU1) it holds true:
\[Q_{1} = \mathrm{\Delta} U_{1} = C_{V}n\left( T_{2}-T_{1}\right) = \frac{3}{2} nR\left( 4 T_{1}-T_{1}\right) = \frac{9}{2} nRT_{1}.\]To calculate Q1, we also use the ideal gas law in the following form
\[nRT_{1} = p_{1}V_{1}\]and we obtain
\[Q_{1} = \frac{9}{2} p_{1}V_{1}.\]Value of CV can be calculated using the Poisson constant and the following formula
\[\kappa = \frac{C_{p}}{C_{V}}\]and Meyer's relation \(C_{p} = C_{V} + R.\)
After the substitution we get
\[\kappa = \frac{C_{p}}{C_{V}} = \frac{C_{V}+R}{C_{V}} = 1+\frac{R}{C_{V}}\, \Rightarrow\] \[\Rightarrow \, C_{V} = \frac{R}{\kappa - 1} = \frac{R}{\frac{5}{3} - 1} = \frac{3}{2}R\]And for Cp it applies
\[C_{p} = \kappa C_{V} = \frac{5}{3}\, \cdot \, \frac{3}{2}R = \frac{5}{2}R.\]During the isobaric cooling, temperature of the gas is lowering, which means that its internal energy is also decreasing, namely, by ΔU2. In addition, the external forces perform work W. Therefore, in agreement with the 1st law of thermodynamics, the gas has to supply the surroundings with heat Q2 (heat Q2 will be negative because it is the heat supplied by the system):
\[Q_{2} = C_{p}n\left( T_{3}-T_{2}\right) = \frac{5}{2} Rn\left( \frac{1}{2}T_{2}- T_{2}\right) = -\frac{5}{4} RnT_{2} \] \[Q_{2} = -\frac{5}{4} p_{2}V_{2} = -5 p_{1}V_{1}\]In the calculation, we have considered the fact that during the isobaric compression, in which volume is reduced to one half, thermodynamic temperature has to decrease to one half too (which follows from the fact that in case of the process at constant pressure \(\frac{V}{T} = const\)). After that we have used the ideal gas law again, this time in the form \(nRT_{2} = p_{2}V_{2}\).
Total heat supplied by the gas during the whole process is given by the sum of heat Q1 received in the isochoric process and heat Q2 supplied by the gas during the isobaric cooling.
It holds true:
\[Q = Q_{1}+Q_{2} =\frac{9}{2} p_{1}V_{1} - 5 p_{1}V_{1}\] \[Q = - \frac{1}{2} p_{1}V_{1}\]Solution: Determining the Change in Internal Energy
Internal energy of the gas is increasing between states 1 and 2 (see figure). Since it is an isochoric process, change in internal energy is equal to the supplied heat.
\[\mathrm{\Delta} U_{1} = Q_{1} = \frac{9}{2} nRT_{1}.\]During the isobaric cooling (process between states 2 and 3), temperature of the gas is lowering, which means that its internal energy is also decreasing. We denote the internal energy change by ΔU2 and calculate it as follows (negative value corresponds with the decrease of internal energy):
\[\mathrm{\Delta} U_{2} = C_{V}n\left( T_{3}-T_{2}\right) = \frac{3}{2} Rn\left( \frac{1}{2}T_{2}- T_{2}\right) = -\frac{3}{4} RnT_{2} \]Note: Change of internal energy does not depend on the type of process that the gas is undergoing. In case of the ideal gas, it depends on temperature change only. Constant of proportionality between the change of internal energy and the temperature change is CV. Supplied or received heat, on the contrary, does depend on the type of process, thus, when calculating the heat, we always have to use the heat capacity corresponding with the process.
We have used the ideal gas law and formulae for the isobaric process again, and then we adjust the relation.
\[\mathrm{\Delta} U_{2}= -\frac{3}{4} p_{2}V_{2} = -3 p_{1}V_{1}\]Now we know all we need to be able to determine the required values. Total increase of internal energy ΔU is given by the formula
\[\mathrm{\Delta} U = \mathrm{\Delta} U_{1} + \mathrm{\Delta} U_{2} = \frac{9}{2} p_{1}V_{1} - 3 p_{1}V_{1}.\] \[\mathrm{\Delta} U = \frac{3}{2} p_{1}V_{1}\]In the calculation, we have considered the fact that during the first process internal energy is increasing and thus, is positive, whereas during the second process internal energy is decreasing, which makes its change negative.
Positive sign of the total change in internal energy means that internal energy of the gas has been increased.
Since internal energy is a state function and therefore its change depends only on the initial and final state, it can be calculated using just the temperature difference T3 − T1:
\[\mathrm{\Delta}U = nC_{V}\left( T_{3} - T_{1} \right)\]For temperature T3 it holds true:
\[T_3 = \frac{1}{2}T_2=\frac{1}{2} 4 T_1 = 2T_1\]and after the substitution we obtain:
\[\mathrm{\Delta}U = nC_{V}\left( 2T_{1} - T_{1} \right) = nC_{V}T_{} = n \left(\frac{3}{2}R\right) T_1 = \frac{3}{2}p_1V_1, \]i.e. the same result as before.
Numerical Substitution
\[W = 2 p_{1}V_{1} = 2\cdot{10^{5}}\cdot{0.01}\, \mathrm{J} = 2000\, \mathrm{J}\] \[W = 2\, \mathrm{kJ}\] \[Q = - \frac{1}{2} p_{1}V_{1} = - \frac{1}{2}\cdot 10^{5}\cdot 0.01\, \mathrm{J} = - 500\, \mathrm{J}\] \[Q = - 0.5\, \mathrm{kJ}\] \[\mathrm{\Delta} U = \frac{3}{2} p_{1}V_{1}= \frac{3}{2}\cdot 10^{5}\cdot 0.01\, \mathrm{J} = 1500\, \mathrm{J}\] \[\mathrm{\Delta} U = 1.5\, \mathrm{kJ}\]Answer
External forces performed the work of 2 kJ, gas supplied the heat of 0.5 kJ and the internal energy of the gas increased by 1.5 kJ.
Comment
Note the validity of the relation ΔU = W + Q. This formula does not state anything else except the compliance of the process with the 1st law of thermodynamics. W is positive, because it is the work done by the external forces, and heat Q is negative because the gas has given it away during the process.