Collection of Solved Problems in Physics

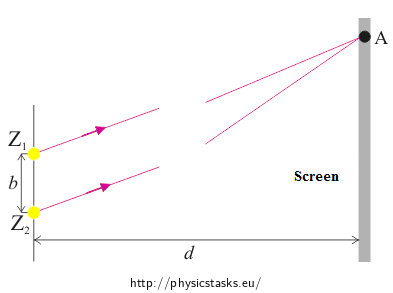
Position of First–Order Maxima When Two Coherent Rays Interfere

Task number: 1699

• Hint 1

  What is the path difference of two rays which are emitted from two sources and incident on the same point of the screen?

• Solution of Hint 1

  We draw a picture where we have two point sources that emit light. The rays are incident on the point A of the screen.

  

  Z₁ and Z₂ are the light sources and d is the distance between the sources and the screen. Now we draw a magnified area of the light sources, and we assume that our screen is far from the optical grating, so that the emitted rays coming to a certain point A of the screen are approximately parallel.

  

  In the picture there is a right-angled triangle from which we can obtain a relation describing the path difference:

  \[\mathrm{\Delta}l = \,b\sin\,\alpha,\]

  where Δl is the path difference of two adjacent rays, b is the distance between the sources and α is the angle between the rays and a central axis.

  Interference maxima or minima can be created in the point A depending on the path difference of two rays.

• Hint 2

  What is the path difference of two rays in order to create an interference maximum?

• Solution of Hint 2

  The rays must create a red interference stripe in the point A, i.e. a maximum. For an interference maximum the following condition must be satisfied:

  \[\mathrm{\Delta}l = k \lambda,\]

  where λ is the wavelength and k is the order of the maximum, therefore k = 0, 1, 2, 3, ...

  In this task we deal with the first-order maximum, i.e. k = 1.

  A more detailed derivation can be found in the task Interference of White Light.

• Hint 3

  What is the relationship between the distance of the first red maximum Δx,which occurs on the screen, and the angle of deflection α?

• Solution of Hint 3

  

  Where d is the distance between the sources and the screen, α is the deviation angle, Δx jis the distance between the first-order red maximum and zero-order maximum.

  The distance Δx scan be expressed using the tangent function. We obtain:

  \[\mathrm{tg}\,\,\alpha=\, \frac{\mathrm{\Delta}x}{d} \quad\Rightarrow\quad \mathrm{\Delta}x=\,d\,\mathrm{tg}\,\,\alpha,\]

  where for α we substitute the relationship evaluated in the previous Hint.

• Given values

  b = 0.45 mm = 4.5·10−4 m	Distance between two light sources
  λ = 700 nm = 7.0·10−7 m	Wavelength of light
  k = 1	Order of maximum
  d = 0.5 m	Distance between the sources and the screen
  Δx = ? (mm)	Distance between the first-order red maximum and the zero-order maximum

• Solution

  The light propagates from its source in all directions. The light from both sources is incident on the screen, and an interference pattern is created. On the screen the interference maxima and minima are formed; their position depends on the path difference of the two rays emitted from the sources.

  Suppose that the screen is far from the sources, so that the rays coming to a point A of the screen are approximately parallel.

  

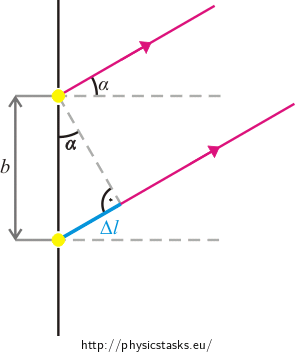
  From the triangle in the picture we obtain a relationship for the path difference Δl of two adjacent rays::

  \[\mathrm{\Delta}l = \,b\sin\,\alpha,\]

  where b is the distance between the sources and α is the angle between the rays and the central axis.

  In the point A the rays interfere and create a stripe, a maximum. Thus the path difference Δl must satisfy the condition:

  \[\mathrm{\Delta}l = k \lambda,\]

  where k = 1. From these two equations we evaluate sin α:

  \[\sin\,\alpha=\,\frac{\lambda}{b}.\]

   

  We draw a picture where we denote d as the distance between the sources and the screen, α as deviation angle, Δx as the distance between the first-order red maximum and the zero-order maximum.

  

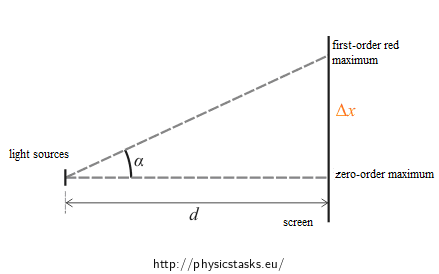
  We obtain:

  \[\mathrm{tg}\,\,\alpha=\, \frac{\mathrm{\Delta}x}{d} \quad\Rightarrow\quad \mathrm{\Delta}x=\,d\,\mathrm{tg}\,\,\alpha,\]

  where α is calculated from the relation:

  \[\sin\,\alpha=\,\frac{\lambda}{b}=\,\frac{7.0{\cdot}10^{−7}}{4.5{\cdot}10^{−4}}\,\dot=\,0.00156 \quad\Rightarrow\quad \alpha\,\dot=\,0.00156,\]

  and we substitute the values given in the assignment.

  \[\mathrm{\Delta}x=\,d\,\mathrm{tg}\,\,\alpha=\,0.5\,\cdot(\mathrm{tg}\,\,0.00156)\,\mathrm{m}\,\dot=\, 8.0{\cdot}10^{−4}\,\mathrm{m}\,\dot= \,0.80\,\mathrm{mm}. \]

   

  Note to the solution:

  To simplify the calculation we can take into account that we deal with very small angles, therefore

  \[\sin\,\alpha\ \dot{=}\ \, \alpha\ \dot{=}\ \mathrm{tg}\,\,\alpha,\]

  where the middle equality is valid if the angle α is in radians.

  Now we solve this task using this finding.

   

  We substitute the relation:

  \[\sin\,\alpha=\,\frac{\lambda}{b},\]

  into the equation for Δx:

  \[\mathrm{\Delta}x=\,d\,\mathrm{tg}\,\,\alpha=\,d\,\frac{\lambda}{b}.\]

  We substitute given values.

  \[\mathrm{\Delta}x=\,d\,\frac{\lambda}{b}=\,0.5\cdot\,\frac{7.0{\cdot}10^{−7}}{4.5{\cdot}10^{−4}}\,\mathrm{m}\,\dot=\,7.8{\cdot}10^{−4}\,\mathrm{m}\,\dot=\,0.78\,\mathrm{mm}.\]

  Thus we can see that the task can be solved with this neglect. This result is not very different from the first result.

• Answer

  The first red stripe appears approximately 0.8 mm from the zero-order maximum.

• Link to experiment

  If you would like to see the interference pattern created on the screen, look at the Double-Slit Experiment.