Imaging a Bat by a Spherical Mirror and a Converging Lens

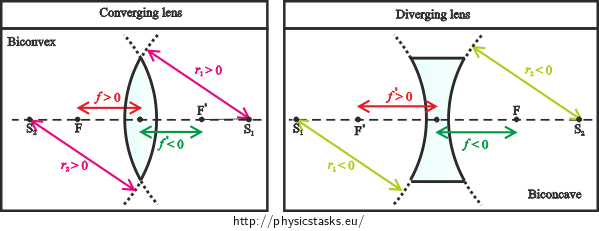
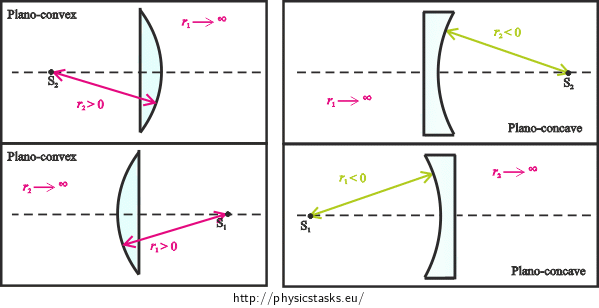
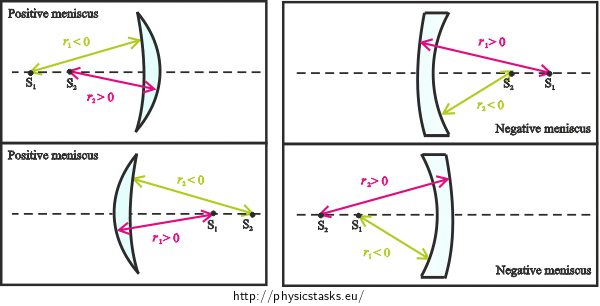
Sign convention for spherical mirrors and lenses

In further explanation we will assume that light beams always travel from the left to the right.

Note: We chose the sign convention as it is stated in grammar school textbooks. You can also encounter a different sign convention, which, however, leads to modification of geometric optics relations. Therefore, it is always necessary to adopt relations along with their sign convention.

Height of the object and the image

We will denote the height of the object by \(y\) and the height of the image by \(y'\). The value of \(y\) or \(y'\) will be positive above the optical axis, and under the optical axis it will be negative.

This applies both to lenses and spherical mirrors.

Object and image distance

Spherical mirrors

Object distance \(a\) will be understood as the distance of the object from the vertex of the mirror.

Object distance \(a\) is always positive.

(We assume the subject is always in front of the mirror.)

Image distance \(a'\) will be understood as the distance of the image from the vertex of the mirror.

Image distance \(a'\) is positive in front of the mirror, and behind the mirror it is negative.

Object and image distance - spherical mirrors

This applies both to concave and convex mirrors.

Lenses

Object distance \(a\) will be understood as the distance of the object from the centre of the lens.

Object distance \(a\) is always positive.

(We assume that the object is always in front of the lens.)

Image distance \(a'\) will be understood as the distance of the image from the centre of the lens.

Image distance \(a'\) is positive in front of the lens, and behind the lens it is negative.

This applies both to converging and diverging lenses.

Radius of curvature and focal length

Spherical mirrors

Radius of curvature \(r\) is positive in front of the mirror and negative behind the mirror.

Focal length \(f\) in front of the mirror is positive and behind the mirror is negative.

Hence, for a concave mirror it holds: \(r\gt0\) a \(f\gt0\).

For a convex mirror, it follows: \(r\lt0\) a \(f\lt0\).

Radius of curvature and focal length - spherical mirrors

Lenses

In case of lenses we denote the radii of curvature by indexes \(1\) and \(2\), where the index \(1\) stands for the optical surface which the beam intersects first.

For convex surfaces, the radii of curvature \(r_{1}\), \(r_{2}\) are positive.

For concave surfaces, the radii of curvature \(r_{1}\), \(r_{2}\) are negative.

For a converging lens, we have \(f\gt0\) and \(f'\lt0\),

where \(f\) is the object focal length and \(f'\) is the image focal length.

For diverging lenses, it holds: \(f\lt0\) and \(f'\gt0\).

Radius of curvature and focal length - lenses 1

Hint 1

Make use of the sign convention and write a notation of the problem.

Solution of Hint 1

Notation

\(y= 4 \mathrm{cm}\)	height of the baby bat

a) Imaging by the spherical mirror
\(y'=−8 \mathrm{cm}\)	height of the image of the baby bat (*)
\(a'= 12 \mathrm{cm}\)	image distance (**)
\(r= ?\)	radius of curvature of the mirror (?)

(*) According to the task assignment, the height of the image is twice as great as the height of the object (\(2{\cdot}4 \mathrm{cm}= 8 \mathrm{cm}\)). The value of the image’s height is negative because the image is inverted and thus is located under the optical axis.

(**) Image distance is positive because the image is in front of the mirror.

(?) According to the sign convention, the radius of curvature is positive for a concave mirror and negative for a convex mirror.

b) Imaging by the converging lens
\(y'= 8 \mathrm{cm}\)	height of the image of the baby bat (***)
\(a'=−12 \mathrm{cm}\)	image distance (****)
\(f= ?\)	focal length of the converging lens (??)

(***) According to the task assignment, the height of the image is twice as great as the height of the object (\(2{\cdot}4 \mathrm{cm}= 8 \mathrm{cm}\)). The value of the image’s height is positive because the image is upright and thus is located above the optical axis.

(****) Image distance is negative because the image is in front of the lens.

(??) According to the sign convention, the focal length of a converging lens is positive.

Hint 2

In both cases (a) and b), we have the specified height of the object \(y \), the height of the image \(y'\) and the image distance \(a'\). We need to find a relation between these variables. Which quantity is represented by the ratio of the image’s height to the height of the object?

Solution of Hint 2

The ratio of the height of the image to the height of the object corresponds to the linear magnification \(Z\): \[ Z=\frac{y'}{y}.\tag{1}\]

Then for the linear magnification \(Z\) it holds: \[ Z=−\frac{a'}{a},\tag{2}\] where \(a\) is the object distance and \(a'\) is the image distance..

By comparison of (1), (2), we obtain the relation for \(y \), \(y'\) and \(a'\): \[ \frac{y'}{y}=−\frac{a'}{a}.\tag{3}\]

Hint 3

Note that the form of the imaging equation is the same for spherical mirror and lenses. Using the imaging equation and relation (3) from the previous hint, determine the focal length \(f\).

Solution of Hint 3

We write the imaging equation: \[\frac{1}{a}+\frac{1}{a'}=\frac{1}{f}.\] We want to express the focal length \(f\). First, we convert fractions on the left side of the equation to the common denominator: \[\frac{a'+a}{a\cdot a'}=\frac{1}{f}.\] Thus: \[f=\frac{a\cdot a'}{a'+a}.\] Factor out \(a\)in the numerator and in the denominator: \[f=\frac{a\cdot a'}{a\left(\frac{a'}{a}+1\right)},\] \[f=\frac{a'}{\frac{a'}{a}+1}.\] Substitute the fraction for \(\frac{a'}{a}\) in accordance with relation (3): \[f=\frac{a'}{\left(−\frac{y'}{y}\right)+1}.\] Adjust the expression: \[f=\frac{a'}{\frac{−y'+y}{y}},\] \[f=\frac{a'\cdot y}{y−y'}.\tag{4}\]

Hint 4

Now we can calculate the focal length of the converging lens. Yet, we still need to find out the relation between the focal length of the spherical mirror \(f\) and its radius of curvature \(r\). Using relation (4), express the radius of curvature \(r\).

Solution of Hint 4

The radius of curvature of a spherical mirror corresponds to twice its focal length: \[r= 2f.\] Substitute \(f\) in accordance with (4): \[r= 2\frac{a'\cdot y}{y−y'}.\tag{5}\]

Hint 5

Write a numerical solution of the problem. You will get the numerical solution of the question a) by substitution values in relation (5), or, in case of question b), in relation (4).

a) In compliance with the result, determine, if the mirror is convex or concave.

b) Is the value of the focal length of the converging lens positive as we expected?

Solution of Hint 5

Numerical solution a) Determining the radius of curvature of the spherical mirror

According to the task assignment and to the sign convention:

\(y= 4 \mathrm{cm}\)

\(y'=−8 \mathrm{cm}\)

\(a'= 12 \mathrm{cm}\)

We substitute for the values in relation (5):

\(r= 2\frac{a'\cdot y}{y−y'}= 2\cdot \frac{12{\cdot} 4}{4−\left(−8\right)} \mathrm{cm}= 2\cdot \frac{48}{12} \mathrm{cm}= 8 \mathrm{cm}.\)

This is a concave mirror because the value of the radius of curvature is positive.

Numerical solution b) Determining the focal length of the converging lens

According to the task assignment and to the sign convention:

\(y= 4 \mathrm{cm}\)

\(y'= 8 \mathrm{cm}\)

\(a'=−12 \mathrm{cm}\)

We substitute for the values in relation (4):

\(f=\frac{a'\cdot y}{y−y'}= \frac{−12{\cdot} 4}{4−8} \mathrm{cm}=\frac{−48}{−4} \mathrm{cm}= 12 \mathrm{cm}.\)

The value of the focal length is positive as we assumed it to be for the converging lens.

Note on Task b)

As we know from the task assignment, the converging lens shows image of the baby bat on the same side as the object and this image is twice as high as the object. Information that the image of the bat is upright is not even necessary. Let us take a look at it.

Let us recall the form of the imaging equation from which we expressed the focal length \( f\): \[f=\frac{a'\cdot y}{y−y'}.\]

Since for a converging lens it holds true that the value of the focal length is positive, the value of the numerator and denominator of the fraction must be both positive (or negative).

Information in the task assignment that the image appears on the same side of the lens as the object tells us that for the image distance it is true that \(a'\lt 0\). The bat standing in front of the mirror is above the optical axis. Thus, the height of the object is positive: \(y\gt 0\). The value of the numerator of the fraction will therefore be negative.

Now we know that the denominator of the fraction has to be negative too. It will be true only if the image of the bat is higher than the bat itself and the height of the image is positive.

Therefore, it must be true that \(\left|y'\right|\gt \left|y\right| \) and at the same time \(y'\gt 0 \).

Overall Solution

Notation

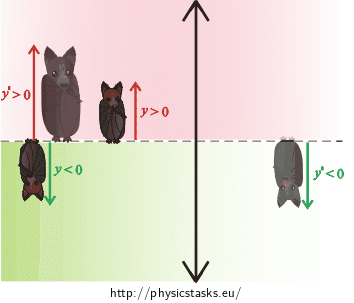
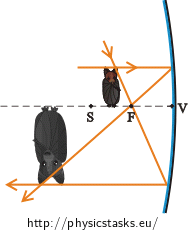
\(y= 4 \mathrm{cm}\)	height of the baby bat

a) Imaging by the spherical mirror
\(y'=−8 \mathrm{cm}\)	height of the image of the baby bat (*)
\(a'= 12 \mathrm{cm}\)	image distance (**)
\(r= ?\)	radius of curvature of the mirror (?)

(*) According to the task assignment, the height of the image is twice as great as the height of the object (\(2{\cdot}4 \mathrm{cm}= 8 \mathrm{cm}\)). The value of the image’s height is negative because the image is inverted and thus is located under the optical axis.

(**) Image distance is positive because the image is in front of the mirror.

(?) According to the sign convention, the radius of curvature is positive for a concave mirror and is negative for a convex mirror.

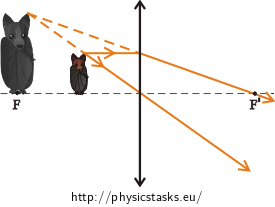
b) Imaging by the converging lens
\(y'= 8 \mathrm{cm}\)	height of the image of the baby bat (***)
\(a'=−12 \mathrm{cm}\)	image distance (****)
\(f= ?\)	focal length of the converging lens (??)

(***) According to the task assignment, the height of the image is twice as great as the height of the object (\(2{\cdot}4 \mathrm{cm}= 8 \mathrm{cm}\)). The value of the image’s height is positive because the image is upright and thus is located above the optical axis.

(****) Image distance is negative because the image is in front of the lens.

(??) According to the sign convention, the focal length of a converging lens is positive.

Ratio of the height of the image to the height of the object

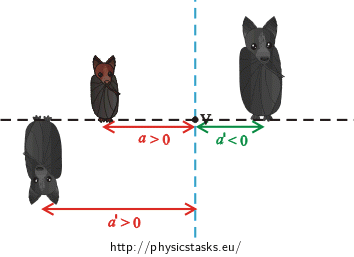
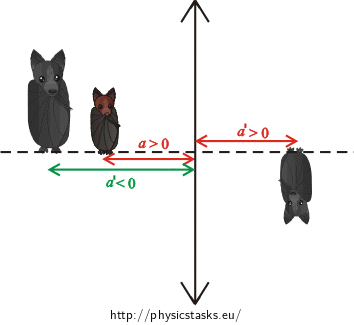
We need to find the relation between the object distance \(a\), image distance \(a'\), height of the object \(y\) and height of the image \(y'\). The ratio of the height of the image to the height of the object corresponds to the linear magnification \(Z\): \[ Z=\frac{y'}{y}.\tag{1}\]

For the linear magnification \(Z\) it is also true that: \[ Z=−\frac{a'}{a},\tag{2}\] where \(a\) is the object distance and \(a'\) is the image distance.

By comparison of (1) with (2) we get the relation between \(y \), \(y'\) and \(a'\): \[ \frac{y'}{y}=−\frac{a'}{a}.\tag{3}\] Now we have prepared a relation that we will use later in the solution.

The imaging equation

Note that the form of the imaging equation is the same both for spherical mirrors and for lenses: \[\frac{1}{a}+\frac{1}{a'}=\frac{1}{f}.\] We want to express the focal length \(f\). First, we convert fractions on the left side of the equation to the common denominator: \[\frac{a'+a}{a\cdot a'}=\frac{1}{f}.\] Thus: \[f=\frac{a\cdot a'}{a'+a}.\] Factor out \(a\) in the numerator and in the denominator: \[f=\frac{a\cdot a'}{a\left(\frac{a'}{a}+1\right)},\] \[f=\frac{a'}{\frac{a'}{a}+1}.\] Substitute for the fraction \(\frac{a'}{a}\) in accordance with relation (3): \[f=\frac{a'}{\left(−\frac{y'}{y}\right)+1}.\] Adjust the expression: \[f=\frac{a'}{\frac{−y'+y}{y}},\] \[f=\frac{a'\cdot y}{y−y'}.\tag{4}\] We have obtained an equation for calculating the focal length of the lens. In case of the spherical mirror, we do not look for the focal length, but for the radius of curvature. Therefore, in the following section, we are going to express the relation for the radius of curvature of the mirror.

Radius of curvature

The radius of curvature of the spherical mirror corresponds to twice its focal length: \[r= 2f.\] Substitute \(f\) in accordance with (4): \[r= 2\frac{a'\cdot y}{y−y'}.\tag{5}\]

Numerical solution

a) Determining the radius of curvature of the spherical mirror

According to the task assignment and to the sign convention:

\(y= 4 \mathrm{cm}\)

\(y'=−8 \mathrm{cm}\)

\(a'= 12 \mathrm{cm}\)

We substitute for the values in relation (5):

\(r= 2\frac{a'\cdot y}{y−y'}= 2\cdot \frac{12{\cdot} 4}{4−\left(−8\right)} \mathrm{cm}= 2\cdot \frac{48}{12} \mathrm{cm}= 8 \mathrm{cm}.\)

This is a concave mirror because the value of the radius of curvature is positive.

b) Determining the focal length of the converging lens

According to the task assignment and to the sign convention:

\(y= 4 \mathrm{cm}\)

\(y'= 8 \mathrm{cm}\)

\(a'=−12 \mathrm{cm}\)

We substitute for the values in relation (4):

\(f=\frac{a'\cdot y}{y−y'}= \frac{−12{\cdot} 4}{4−8} \mathrm{cm}=\frac{−48}{−4} \mathrm{cm}= 12 \mathrm{cm}.\)

The value of the focal length is positive as we assumed it to be for the converging lens.

Answer

a) The radius of curvature of the spherical mirror is \( 8 \mathrm{cm}\). The mirror is concave.

b) The focal length of the converging lens is \( 12 \mathrm{cm}\).

How far in front of the lens or mirror is the bat?

We are looking for the object distance \(a\). In both cases, we use the relation: \[ \frac{y'}{y}=−\frac{a'}{a}\tag{3}\] and express the object distance from it: \[ a=−\frac{y}{y'}a'.\]

The spherical mirror

\(y= 4 \mathrm{cm}\)

\(y'=−8 \mathrm{cm}\)

\(a'= 12 \mathrm{cm}\)

Substitution:

\( a=−\frac{y}{y'}a'=−\frac{4}{\left(−8\right)}\cdot 12 \mathrm{cm}=6 \mathrm{cm}.\)

The converging lens

\(y= 4 \mathrm{cm}\)

\(y'= 8 \mathrm{cm}\)

\(a'=−12 \mathrm{cm}\)

Substitution:

\( a=−\frac{y}{y'}a'=−\frac{4}{8}\cdot \left(−12\right) \mathrm{cm}=6 \mathrm{cm}.\)

We see that the bat is in the distance of \(6 \mathrm{cm}\) in front of the spherical mirror or lens.

Collection of Solved Problems in Physics