Field of Thick Charged Plate
Task number: 1533
An infinite plate of a thickness a is uniformly charged with a charge bulk density ρ
a) Find the electric field intensity at a distance z from the centre of the plate.
b) Also determine the electric potential at a distance z from the centre of the plate.
Consider a field inside and outside the plate.
Hint: Electric field intensity
To solve this task we will use Gauss's law, it is therefore required to choose a Gaussian surface.
A suitable surface is a surface of a cylinder whose axis is perpendicular to the plate and the plate centre passes through the centre of the cylinder. Due to the symmetry of the charge distribution, the vector of electric intensity is perpendicular to the plate, and is therefore perpendicular or parallel to the individual parts of the selected Gaussian surface (see How to choose a Gaussian surface? in the task Pole rovnoměrně nabité koule), which simplifies the calculation of electric flux.
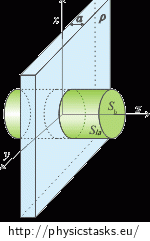
We divide the task into two parts:
- The length of the Gaussian cylinder is greater than the thickness of the plate.
- The length of the Gaussian cylinder is smaller than the thickness of the plate.
Hint: Electric potential
Potential is potential energy per unit charge:
\[\varphi\,=\, \frac{E_p}{Q}\]and potential energy is equal to negative taken work done by electric force when transferring a unit charge from a point of zero potential energy to a given point.
\[E_p(z)\,=\, - \int^z_{0} \vec{F} \cdot \mathrm{d}\vec{z}\]Zero potential energy is chosen in the centre of the plate. In this case we cannot choose the zero potential energy in infinity, as usual, because the integral would have an infinite value at all points. This behaviour is caused by the infinite length of the plate, i.e. the charged volume "reaches" to infinity.
We divide both sides of the equation by charge Q.
\[\varphi\,=\, - \int^z_{0} \frac{\vec{F}} {Q}\cdot \mathrm{d}\vec{z}\]Force \(\vec{F}\) divided by charge Q, is the electric field intensity \(\vec{E}\).
\[\varphi\,=\, - \int^z_{0} \vec{E}\cdot \mathrm{d}\vec{z}\]Hint: Potential outside the plate
Attention! We calculate the work done by electric force when transferring a charge from the centre of the plate. The electric intensity, which is substituted into the integral, is not expressed by the same relation along the path of integration; for calculating the work outside and inside the plate we need to use different relations. This requires the integral in the expression for the potential to be divided into two integrals.Analysis
We divide this task into two parts. We examine the field outside and inside the plate separately.
Due to symmetrical charge distribution, the easiest way to find the intensity of electric field is using Gauss's law. Gauss's law relates the electric flux through a closed area and the total charge enclosed in this area.
The vector of electric intensity is perpendicular to the plane of the plate at all points and its magnitude depends only on the distance from the centre of the plate. (Depending on the sign of the charge the vector either points towards or outwards the plate.) This is due to the symmetrical distribution of the charge. Due to this symmetry we can also solve the whole task only for positive z values, the only thing that changes for negative z is the direction of the vector of electric intensity.
We choose the Gaussian surface to be a surface of a cylinder with its axis perpendicular to the plate, and the centre of the plate passes through the centre of the cylinder. In such a case, the vector of electric field intensity is perpendicular to both bases of the cylinder and it is also parallel to the lateral area of the cylinder. Thus we simplify the calculation of the intensity flux.
The total flux through the Gaussian surface is obtained by summing the flux through the lateral area and the bases of the cylinder.
When calculating the intensity inside the plate, the length of the cylinder is smaller than the thickness of the plate. A charge closed inside the cylinder is given by the volume of this cylinder.
When calculating the intensity outside the plate, the cylinder length is greater than the thickness of the plate. A charge closed inside this surface is given by a volume of a cylinder of the same base as the Gaussian cylinder and of length equal to the thickness of the plate.
Potential at a given point is equal to a negative taken integral of intensity from a point of zero potential to the given point. Zero potential is selected in the centre of the plate. (A more detailed explanation is given in the section Hint.)
When calculating the potential outside the plate we must take into account that the electric field intensity is not described by the same relation along the path of integration. The relations describing the intensity outside and inside the plate differ. Therefore, we must first determine the work that is needed to transfer a unit charge from the centre of the plate to the plate surface and the work required for transferring the charge away from the plate.
Solution: Intensity outside the plate
In this section we determine the intensity of the electric field outside the plate, i.e. for z > a/2.
We use Gauss's law:
\[\oint_S \vec{E} \cdot \mathrm{d}\vec{S}\,=\, \frac{Q}{\varepsilon_0}\] \[\oint_S \vec{E} \cdot \vec{n}\mathrm{d}S\,=\, \frac{Q}{\varepsilon_0}\]Due to the symmetric distribution of the charge within the plate, the electric field around the plate is also symmetric. The vector of electric field intensity is perpendicular to the plate at all points and its magnitude depends only on the distance from the centre of the plate.
We choose the Gaussian surface to be a surface of a cylinder with its axis perpendicular to the charged plate, the centre of the plate passes through the centre of the cylinder. The surface is chosen this way, because the vector of electric intensity is perpendicular to the cylinder bases and parallel to the lateral area of the cylinder, which simplifies the calculation of the scalar product. (General procedure How to choose a Gaussian surface is described in the task Pole rovnoměrně nabité koule.)
The total intensity flux through the cylinder is obtained by summing the flux through the cylinder bases and the flux through the lateral area of the cylinder. Since the two cylinder bases at the same distance from the charged plate, the electric intensity vector is on both bases of the same magnitude. The electric flux through these bases is also the same; therefore we take the flux through one base of the cylinder twice. We adjust the left side of Gauss's law:
\[2\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,+\,\oint_{S_la} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\, \frac{Q}{\varepsilon_0}\tag{*}\]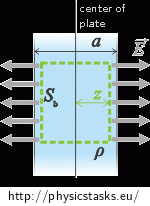
Flux through lateral area:
The vector of electric intensity is parallel to the lateral area of the cylinder; hence it is perpendicular to the normal vector. Therefore the scalar product of these vectors is equal to zero, which results in zero flux through the lateral area.
\[\oint_{S_la} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\,0\]Flux through base:
The vector of electric intensity is perpendicular to the cylinder base at all points, and thus parallel to a normal vector. We can simplify the scalar product.
\[ \vec{E} \cdot \vec{n} \,=\, En\,=\,E\](Note: \(\vec{n}\) is a unit vector).
Using these findings we can adjust the integral on the left side of Gauss's law and evaluate the flux through the cylinder base.
\[\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\,\oint_{S_b} E n\mathrm{d}S\,=\, \oint_{S_b} E\mathrm{d}S\]The vector of electric field intensity E is of the same magnitude at all points of the chosen surface, so we can factor it out of the integral as a constant. We obtain a relation:
\[\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\,E \oint_{S_b} \mathrm{d}S\,=\,ES_p\,,\]where Sb is the surface area of the base of Gaussian cylinder.
The resulting formula is substituted back into Gauss's law (*). We must not forget that there are two bases of the Gaussian cylinder, so we multiply the flux through the cylinder base by two.
\[2 E S_b\,=\, \frac{Q}{\varepsilon_0}\]Now we evaluate the charge Q inside the Gaussian surface. The Gaussian cylinder contains a part of the plate, which is shaped like a cylinder with the base Sb and the height a. We express the charge by using this volume and the charge density Q = ρV = ρaSp.
\[2 E S_b\,=\, \frac{\varrho a S_b }{\varepsilon_0}\]We divide the equation by Sb
\[2 E \,=\, \frac{\varrho a}{\varepsilon_0}\]And we evaluate the intensity of electric field of the charged plate.
\[E \,=\, \frac{\varrho a}{2 \varepsilon_0}\]We have derived that the magnitude of electric intensity does not depend on the distance z from the charged plates. The electric field of a charged plate is uniform.
Solution: Intensity inside the plate
In this section we determine the electric field intensity inside the charged plate, i.e. for z < a/2. The procedure is similar to that in the previous section, so it is not commented in detail.
We use Gauss's law:
\[\oint_S \vec{E} \cdot \vec{n}\mathrm{d}S\,=\, \frac{Q_1}{\varepsilon_0}\,,\]where Q1 is the charge inside the Gaussian surface.
We choose the Gaussian surface to be a surface of a cylinder with length 2z, its axis being perpendicular to the plate and the centre of the plate passes through the centre of the cylinder. The total electric flux is obtained by adding the flux through the lateral area and through both bases of the cylinder.
\[2\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,+\,\oint_{S_la} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\, \frac{Q}{\varepsilon_0}\tag{*}\]
Electric flux through lateral area:
The vector of electric intensity is parallel to the lateral area of the cylinder; hence the electric flux is zero.
Electric flux through bases:
The vector of electric intensity is perpendicular to the cylinder base at all points, and thus parallel to a normal vector. We can simplify the scalar product.
\[ \vec{E} \cdot \vec{n} \,=\, En\,=\,E\](Note: \(\vec{n}\) is a unit vector).
and adjust the integral on the left side of Gauss's law
\[\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\,\oint_{S_b} E n\mathrm{d}S\,=\, \oint_{S_b} E\mathrm{d}S\,=\,E \oint_{S_b} \mathrm{d}S\]The integral is equal to a surface area of the cylinder base.
\[\oint_{S_b} \vec{E} \cdot \vec{n}\mathrm{d}S\,=\,E S_b\]The resulting formula is substituted back into Gauss's law (*). We must not forget that the cylinder has two bases, so we multiply the flux through one base by two.
\[2 E S_b\,=\, \frac{Q_1}{\varepsilon_0}\]Now we evaluate the charge Q1 inside the Gaussian surface. The Gaussian cylinder contains a part of the plate, which is shaped like a cylinder with the base Sb and the height 2z. We express the charge using this volume and the charge density Q1 = ρV = 2zρSb.
\[2 E S_b\,=\, \frac{ 2z \varrho S_b }{\varepsilon_0}\]We divide the equation by Sb:
\[E \,=\, \frac{\varrho}{\varepsilon_0} \,z\]Solution: Potential inside the plate
Potential at a distance z from the centre of the plate at point A is equal to negative taken integral of intensity from a point of zero potential to point A. Zero potential is selected in the centre of the plate. (A more detailed explanation is given in Hint.)
\[\varphi (z)\,=\, - \int_{0}^z \vec{E} \cdot \mathrm{d}\vec{z}\]Note: If we choose zero potential in infinity, as we do in the majority of the tasks, we cannot calculate the integral.
The potential does not depend on the choice of the integration path so the path can be selected at will. In this task we choose the path to be a part of a straight line which is perpendicular to the plate. The vector of electric field intensity \(\vec{E}\) is parallel to the \(\vec{z}\) vector. Therefore, we can simplify the integral.
\[ \varphi (z)\,=\, - \int^{z}_{0} E \mathrm{d}z \]Now we divide the task into two separate cases and we calculate the potential inside and outside the plate separately.
First, we evaluate the potential at a distance z from the centre of the plate inside the plate, i.e. z < a/2. We substitute the electric intensity E derived in the previous section.
\[ \varphi (z)\,=\, - \int^{z}_{0}\frac{\varrho}{\varepsilon_0}\,z \mathrm{d}z \]We factor the constants out of the integrals and calculate the integrals.
\[ \varphi (z)\,=\, - \frac{\varrho}{\varepsilon_0}\int^{z}_{0}z \,\mathrm{d}z \,=\, -\,\frac{\varrho}{\varepsilon_0} \left[ \frac{z^2}{2}\right]^{z}_{0} \,=\, -\,\frac{\varrho}{\varepsilon_0}\, \frac{z^2}{2}\,.\]The potential inside the plate is represented by the formula
\[ \varphi (z)\,=\, -\,\frac{\varrho}{\varepsilon_0}\, \frac{z^2}{2}\,.\]Solution: Potential outside the plate
When calculating the potential outside the plate (i.e. for z > a/2), we proceed similarly as in the previous section. We evaluate the potential from this equation:
\[\varphi (z)\,=\, - \int_{0}^z \vec{E} \cdot \mathrm{d}\vec{z}\,=\, - \int_{0}^z E \mathrm{d}z\,.\]When evaluating the potential we have to take into account that the formulae describing the electric intensity differ along the path of integration. The point, where we need to use the second relation, is the surface of the plate. The integral must therefore be divided into two parts. First, we have to transfer the charge to the surface of the plate (i.e. to a distance a/2 from the centre of the plate), and then from the plate surface further into the plate.
\[\varphi (z)\,=\, - \int^{\frac{a}{2}}_{0} E_{in}\,\mathrm{d}z \, -\, \int^{z}_{\frac{a}{2}} E_{out}\,\mathrm{d}z\]We substitute the electric intensity that we have evaluated in previous sections.
\[\varphi (z)\,=\, - \int^{\frac{a}{2}}_{0} \frac{\varrho}{\varepsilon_0}\,z \,\mathrm{d}z -\, \int^{z}_{\frac{a}{2}}\frac{\varrho a}{2 \varepsilon_0} \,\mathrm{d}z \,\]We factor all constant out of the integrals and we calculate the integrals.
\[\varphi (z)\,=\, - \frac{\varrho}{\varepsilon_0}\int^{\frac{a}{2}}_{0}z \mathrm{d}z\, -\, \frac{\varrho a}{2 \varepsilon_0}\int^{z}_{\frac{a}{2}} \mathrm{d}z \,=\,-\,\frac{\varrho}{\varepsilon_0} \left[ \frac{z^2}{2}\right]^{\frac{a}{2}}_{0}\, - \,\frac{\varrho a}{2 \varepsilon_0}\left[z\right]^{z}_{\frac{a}{2}}\]Note: We did not have to calculate the first integral, we could have only substituted z = a/2 into the result of the previous section..
We add corresponding components together.
\[\varphi (z)\,=\, - \,\frac{\varrho a^2}{8 \varepsilon_0} \, -\,\frac{\varrho}{2 \varepsilon_0}\, z^2\, +\,\frac{\varrho a^2}{4\varepsilon_0}\] \[\varphi (z)\,= \,\frac{\varrho a^2}{8 \varepsilon_0} \, -\,\frac{\varrho}{2 \varepsilon_0}\, z^2\, \]We have evaluated electric potential outside the charged plate.
Answer
Electric field generated around a charged plate is uniform with the electric intensity
\[E \,=\, \frac{\varrho a}{2 \varepsilon_0}\,.\]The intensity inside the plate is given by
\[E \,=\, \frac{\varrho}{\varepsilon_0} \,z\,.\]The direction of electric intensity vector is either outwards or towards the plate, depending on the sign of the charge.
The potential outside the plate is
\[\varphi (z)\,=\,\frac{\varrho a^2}{8 \varepsilon_0} \, -\frac{\varrho a}{2 \varepsilon_0}\,|z| \,.\]The potential inside the plate is
\[\varphi (z)\,=\, -\,\frac{\varrho}{2 \varepsilon_0}\, z^2\,. \]Note: In these relations we see z as a coordinate, not as a distance from the plate; that is why we added the absolute value.
Graphs
We consider the plate to be charged with a positive charge.
Graph of electric intensity as a function of a distance from the centre of the plate:
Due to the symmetry of the electric field intensity, the graph is also symmetric with respect to the origin. To show that the vector of electric intensity changes direction in the centre of the plate, the electric intensity reaches negative values after crossing the centre of the plate.
The electric field is uniform outside the plate with intensity \(E\,=\, \frac{\varrho a}{2 \varepsilon_0}\).
Thus, the function over the interval a/2 to infinity is constant. For negative values of z the function is negative.
The intensity inside the plate is given by \(E\,=\, \frac{\varrho}{ \varepsilon_0} z\).
The electric intensity increases linearly inside the plate from the centre to the surface of the plate. The intensity in the centre of the plate is zero.

The function is continuous. This can be proved by substituting z = a/2 into both relations describing the electric intensity.
Note: The electric field intensity is continuous, except for points where it passes through a charged surface. Only the tangent components of the vector of electric intensity remain continuous in this case. The normal component changes "by steps" proportional to the surface charge density. In this task, however, there are no charged surfaces.
Graph of electric potential as a function of a distance from the centre of the plate:
The electric potential outside the charged plate is \(\varphi (z)\,=\,\frac{\varrho a^2}{8 \varepsilon_0}- \, \frac{\varrho a}{2 \varepsilon_0}|z|.\)
The potential inside the plate is \(\varphi (z)\,=\,-\,\frac{\varrho}{2 \varepsilon_0}\,z^2\,.\)
For z in the interval from (-a/2 to a/2) the graph is parabola with its vertex in the origin (i.e. in the centre of the plate). Outside the plate the potential decreases linearly.

The function is at the points z = a/2 and z = −a/2 continuous.
Moreover, the first derivatives of this function are at these points also continuous, therefore the function is smooth.
Note: The electric potential is always continuous, because it is actually work done by transferring a unit charge and it can not be changed "by steps". With the exception of points on charged surfaces, the first derivative of the potential is also continuous, i.e. the potential is a smooth function.
Calculation of intensity using intensity around a thin plate
The task Pole rovnoměrně nabité roviny describes the electric intensity around a thin plate. We can use this result to solve this task. We cut the plate into thin plates with area charge density Δσ = ρΔr.
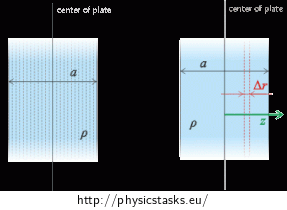
Each thin plate generates a uniform field with intensity
\[\mathrm{\Delta} E\,=\, \frac{\mathrm{\Delta} \sigma}{2 \epsilon_0}\,=\, \frac{\varrho \mathrm{\Delta} r}{2 \epsilon_0}\,.\]To get the overall intensity at a distance z > a/2 from the centre of the thick plate, we add contributions of all these thin plates.
\[E\,=\, \int^{\frac{a}{2}}_{-\frac{a}{2}} \frac{\varrho }{2 \epsilon_0}\,\mathrm{d} r\]We factor constants out of the integral and we calculate the definite integral.
\[E\,=\,\frac{\varrho }{2 \epsilon_0} \int^{\frac{a}{2}}_{-\frac{a}{2}}\mathrm{d} r\,=\, \frac{\varrho }{2 \epsilon_0}[r]^{\frac{a}{2}}_{-\frac{a}{2}}\,=\, \frac{\varrho }{2 \epsilon_0}\,\left(\frac{a}{2}+\frac{a}{2}\right)\] The plate generates a uniform electric field: \[E\,=\, \frac{\varrho a }{2 \epsilon_0}\]We obtain the same result as when using Gauss's law.
Intensity inside the plate:
We have to add the contributions from the plates on the left and right sides from the point where we investigate the electric intensity. The vectors of electric intensity do not have the same direction. The intensity vector on the left side points to one side, on the right side it points to the other side. We therefore have to subtract the contributions:
\[E\,=\, \int^{z}_{-\frac{a}{2}} \frac{\varrho }{2 \epsilon_0}\,\mathrm{d} r\,-\,\int^{\frac{a}{2}}_{z} \frac{\varrho }{2 \epsilon_0}\,\mathrm{d} r\]We factor the constants out of the integrals and we calculate the integrals.
\[E\,=\, \frac{\varrho }{2 \epsilon_0}\int^{z}_{-\frac{a}{2}}\mathrm{d} r\,-\, \frac{\varrho }{2 \epsilon_0}\int^{\frac{a}{2}}_{z}\mathrm{d} r \,=\, \frac{\varrho }{2 \epsilon_0}[z]^{z}_{-\frac{a}{2}}\,-\, \frac{\varrho }{2 \epsilon_0}[z]^{\frac{a}{2}}_{z}\] \[E\,=\, \frac{\varrho }{2 \epsilon_0}(z\,+\, \frac{a}{2}\,-\, \frac{a}{2}\,+\,z)\,=\, \frac{\varrho }{2 \epsilon_0}\,2z\]The electric field intensity inside the thick plate at a distance z from the centre of the plate is
\[E\,=\, \frac{\varrho }{\epsilon_0}\,z\,,\]which is the same result that we obtained when using Gauss's law.
Link to a similar task
The task Pole rovnoměrně nabité roviny deals with a very thin plate, which makes the calculations simpler.



