Electron in the screen of oscilloscope
Task number: 548
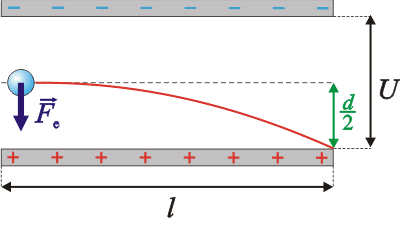
An electron was accelerated by voltage 600 V and flew into the middle of deflection plates of a screen. The distance between plates is 4 mm and their length is 16 mm.
What voltage is needed for the electron to fall at the end of the deflection plate?
Hint 1
Draw a figure of the whole situation. Which force affects the electron flying between both plates?
What movement is it?
Picture
The trajectory of the electron between the deflection plates is marked by the red line.
Note: We ignore the gravitational force during the solution of the task because it is very small compared with the electricity force (see the comment at the end of task).
Hint 2
We can express acceleration of the electron by applying Newton’s second law in which we put the electric force. The electric force is directly proportional to the electric field and the charge of the electron.
Hint 3
Power supply performs work during the acceleration of the electron so it gives it kinetic energy. The work depends on the voltage of the power supply and the charge of the electron.
Analysis
Power supply performs work during the acceleration of the electron with voltage U so the electron gains kinetic energy. We can find the velocity of the electron, which has flown between the deflection plates, from this kinetic energy.
When the electron flies between the deflection plates, the electric force will affects it. The direction of the electric force is perpendicular to the movement of the electron (see the figure). The situation is the same as when we throw for example a ball in the gravitational field. So, the trajectory of the electron will be the same as during the horizontal throw, i.e. the trajectory is a parabola.
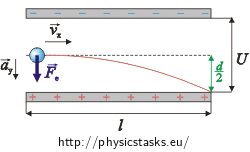
We can divide the movement of the electron into two parts:
- Horizontal movement with constant velocity, which the electron had when it was flying between the plates.
- Vertical movement with constant acceleration, which is caused by the electric force.
We can find out how long the electron will be moving until it falls at the end of the deflection plate. This duration we put to relation for the distance ofthe movement with constant acceleration.
Acceleration of the electron moreover plays a role in relation for the distance. We can express it using an electric force and Newton’s second law. Electric force is directly proportional to the electric field and the charge of the electron.
Electric field rises with voltage on the deflection plates and falls with the distance between them.
Solution: Velocity of the Electron When It Flies Between the Plates
Power supply performs work during the acceleration of the electron with voltage U so the electron gains kinetic energy. The power supply, which accelerates the electron, performs work work:
\[W\,=\,QU\,.\]Because we accelerate electron, we can substitude the charge of the electron:
\[W\,=\, eU\,.\]So, the electron gains kinetic energy:
\[E_k\,=\, \frac{1}{2} m v_x^2\,,\]where m is the mass of the electron and vx is the velocity of the electron after acceleration (and before the electron will have flown between the plates).
We can express velocity vx of the electron, which has flown between the deflection plates, from these two equations.
\[E_k\,=\, W\] \[\frac{1}{2} m v_x^2\,=\, eU\] \[v_x^2\,=\, \frac{2eU }{m}\] \[v_x\,=\,\sqrt{ \frac{2eU }{m}}\]Solution: Acceleration of the Electron in Vertical Direction
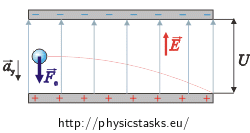
Electric force Fe affects the electron in vertical direction. We can express this force using the charge of th electron and electric field.
\[F_e \,=\, e E\]
An uniform electric field between charged plates is
\[E\,=\, \frac{U}{d}\,.\]So, we can express electric force as
\[F_e \,=\, \frac{ e U}{d}\,.\tag{*}\] Now we can find acceleration, which affects the electric force, from Newton’s second law. \[a_y\,=\, \frac{F_e}{m}\] When we substitute the equation (*) for the electric force, we gain relation for acceleration \[a_y\,=\, \frac{\frac{ e U}{d}}{m}\] \[a_y\,=\, \frac{ e U}{md }\]Solution: Voltage Between Plates
When the electron flies between the plates, electric force Fe will affect it in the vertical direction.
We can divide the movement of the electron into two parts. Electron flies horizontally with constant velocity vx and simultaneously in a vertical direction with constant acceleration ay.
Now we express the distance, which the electron will cover in horizontal and vertical directions.
The electron will fly in the horizontal direction across the whole plate of length ll
\[l\,=\, v_x t\]During the same time intervalt the electron will fly with constant acceleration and it will cover half the distance from plate to plate, i.e. distance d/2
. \[\frac{d}{2}\,=\,\frac{1}{2}a_y t^2\]We express time t from the first equation
\[t\,=\, \frac{l}{v_x}\]and substitude it into the second equation
\[\frac{d}{2}\,=\,\frac{1}{2}a_y \frac{l^2}{v_x^2}\,.\]Now replace velocity vx and acceleration ay with relations, wehave expressed in the previous sections.
\[\frac{d}{2}\,=\,\frac{1}{2}\, \frac{e U}{m d}\, \frac{l^2}{\frac{2 U_o e}{m}}\] We will edit the equation so that we gain the voltage U. \[d\,=\,\, \frac{ U}{ d}\, \frac{l^2}{2 U_0 }\] \[U\,=\,\, \frac{2 U_0 d^2}{l^2}\]Comment: If the voltage ay was bigger, the electric force would be bigger too and the electron would fall on the plate before it reaches its end. If the voltage was smaller, the electron would be deflected from the horizontal direction but not too much. So, the electron would fly through between the plates.
Numerical Values
U0 = 600 V
d = 4 mm = 4·10−3 m
l = 16 mm = 1,6·10−2 m
U = ? (V)
\[U\,=\,\, \frac{2 U_0 d^2}{l^2}\,=\,\frac{2 \cdot\, 600 \cdot\, \left( 4 {\cdot} 10^{-3}\right)^2}{\left(1{,}6 {\cdot} 10^{-2}\right)^2}\,\mathrm{V}\] \[U\,=\,75\,\mathrm{V}\]Answer
We need voltage so that the electron would fall at the end of the deflection plate \[U\,=\,\, \frac{2 U_0 d^2}{l^2}\,=\,75\,\mathrm{V}\,.\]
TV Screen
Deflection of the rays of electrons using deflection plates is used for example in screens of oscilloscopes. The deflection angle is relatively small therefore the screen of oscilloscope is relatively small and long.
In TV screens the rays is deflected because of the use of the magnetic field of system of coils (the principle is the same as deflection of α particles in task Urychlená α částice (only in Czech)).
(Comment: Plasma and LCD screens use another principle.)
Comparison of Electric and Gravitational Forces
We can calculate both forces from equations:
\[F_e\,=\,\frac{eU}{d}\] \[F\,=\,mg\,.\]Substitude numerical values of quantities.
e = 1,6·10−19 C
U = 75 V
d = 4·10−3 m
m = 9,1·10−31 C
Gain
\[F_e\,=\,\frac{1{,}6{\cdot} 10^{-19}\,\cdot\,75}{4{\cdot} 10^{-3}}\,\mathrm{N}\,=\,3{\cdot} 10^{-15}\,\mathrm{N}\] \[F\,=\,9{,}1{\cdot} 10^{-31}\,\cdot\,10\,\mathrm{N}\,=\,9{,}1{\cdot} 10^{-30}\,\mathrm{N}\]Gravitational force is smaller by fifteen orders of magnitude so we can ignore it in this task.
If we couldn’t or didn’t want to ignore it, we would calculate with the fact that there is a constant force affecting the electron in vertical direction with intensity Fe + FG. Thus, the task wouldn´t be too much complicated either.
