Collection of Solved Problems in Physics

Magnetic Field of a Straight Conductor Carrying a Current

Task number: 1786

• Hint 1

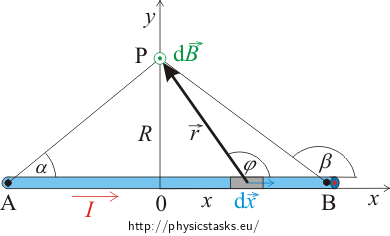
  Draw a picture of the straight conductor with the current and choose and arbitrary point P at a distance of R from the conductor. Segment the conductor into short elements. Every element of the conductor contributes to the magnetic field at point P. The total magnetic field at point P is equal to the sum of the contributions from all elements of the conductor. Making the elements very (infinitely) short, we proceed from summation to integration of the contributions to the magnetic field from inidividual parts of the conductor.

  Draw the directions of the contributions to the B-field of several elements of the conductor into the picture and determine the direction of the total magnetic B-field.

• Solution of Hint 1

  The figure below shows the conductor carrying the current. We labeled the ends of the conductor A and B. The positions of the end points A, B relative to point P are determined by angles α and β.

  

  We put the origin of the coordinate system at the foot of the perpendicular from point P to the conductor. Each element of the conductor makes a contribution \(\mathrm{d}\vec{B}\) do the magnetic B-field at point P, which is given as

  \[\mathrm{d}\vec{B} = \frac{\mu_o}{4\pi}\, \frac{I\, \mathrm{d}\vec{x}\times \vec{r}}{r^{3}}.\]

  The direction of vector \(\mathrm{d}\vec{B}\) is given by the right-hand rule. Contributions from all conductor elements aim in the same direction - out of the picture plane. The resulting vector \(\vec{B}\) of the magnetic field thus also aims towards us.

  The amplitude of the total magnetic B-field at point P is then given by an integral over elements of the conductor, i.e. over the whole length of the conductor.

• Hint 2

  We have already said in Hint 1 that we segment the conductor into very short pieces. Each of these pieces contributes to the total magnetic field at poin P. We calculate the total magnetic B-field at point P by integrating over all contributions dB to the field at point P.

  Our task is to express all quantities that change when we move along the conductor by using only one variable. The integral is the simplest when we choose the angle formed by the conductor and the connecting line from the conductor element to point P for this variable. Think over, what other quantities that appear in the formula of the magnetic field contribution dB do change when we move along the conductor. Express these quantities using the angle and integrate over the span of the angle.

• Hint solution — Expressing the variables

  The figure depicts the conductor carrying the current with ends labelled A and B. We have said in the hint that the integral has the simplest form when we choose the angle between the vector \(\vec{r}\) and the vector \(\mathrm{d}\vec{x}\) to be the integration variable. The interval of integration is delemited by the angles α and β of points A and B, respectively, as seen from point P.

  

  The quantities that change during integration are the distance r from the condcutor element to point P, position x of the element and angle φ. Let us express all these variables using the angle φ (see the figure). The distance r is given by:

  \[r=\frac{R}{\sin{\varphi}}.\]

  This relation is more apparent for elements on the left part of the conductor, yet the sine function is defined also for angles above 90°.

  For the distance r it holds that

  \[x=\frac{R}{tg(\pi -\varphi)}=R\,\mathrm{cotg}\,(\pi -\varphi)=-R\,\mathrm{cotg}\,\varphi\]

  and differentiating of this substitution gives us the relation between the differentials:

  \[\mathrm{d}x=\frac{R}{\sin^2{\varphi}}\,\mathrm{d}\varphi.\]

• Analysis

  We assess the magnitude of the B-field at any point P outside of the straight conductor carrying the currnet by using the Biot-Savart law.

  We consider contributions to the magnetic field at point P from all elements of the straight conductor. The total magnitude of the magnetic B-field at point P is given by an integral over all elements of the conductor

  We just shift the endpoints A and B of the conductor to infinity to get the magnitude of the B-field from an infinitely long straight conductor at point P.

• Solution 1

  We assess the contributions \(\mathrm{d}\vec{B}\) from individual elements of the straight conductor carrying the current using the Biot-Savart law that states:

  \[\mathrm{d}\vec{B} = \frac{\mu_o}{4\pi}\, \frac{I\, \mathrm{d}\vec{x}\times \vec{r}}{r^{3}}.\]

  The absolute value of the vector product is

  \[|\mathrm{d}\vec{x}\times\vec{r}|=\mathrm{d}x\,r\,\sin{\varphi},\]

  where φ is the angle formed by the vectors \(\mathrm{d}\vec{x}\) and \(\vec{r}\).

  We have argued in the hint that all the contributions aim in the same direction. We thus get the resulting magnitude of the B-field by integrating over all the contributions dB.

  \[B=\int_A^B \mathrm{d}B = \frac{\mu_o}{4\pi}\, \int_A^B\frac{I\, \mathrm{d}x\,r\,\sin{\varphi}}{r^{3}}=\frac{\mu_o}{4\pi}\,I\int_A^B\frac{\sin{\varphi}}{r^{2}}\,\mathrm{d}x.\tag{1}\]

  Variables r, x and φ change on integrating over the length of the conductor. We express r and x as functions of φ. The distance r is

  \[r=\frac{R}{\sin{\varphi}},\]

  which is better apparent in the left section of the figure, yet the sine function is defined also for obtuse angles. It further holds that

  \[x=\frac{R}{\mathrm{tg}\,{(\pi -\varphi)}}=R\,\mathrm{cotg}\,{(\pi -\varphi)}=-R\,\mathrm{cotg}\,{\varphi}\]

  and we get by differentiating that

  \[\mathrm{d}x=\frac{R}{\sin^2{\varphi}}\,\mathrm{d}\varphi.\]

  

  We substitute for the variables in equation (1)

  \[B= \frac{\mu_o}{4\pi}\,I \int_\alpha^\beta \frac{R}{\sin^2{\varphi}}\,\frac{\sin^2{\varphi}}{R^2}\sin{\varphi}\,\mathrm{d}\varphi.\]

  Which yields the equation for calculating the resulting magnetic B-field:

  \[B= \frac{\mu_o}{4\pi}\,\frac{I}{R} \int_\alpha^\beta \sin{\varphi}\,\mathrm{d}\varphi.\]

  We integrate:

  \[B= \frac{\mu_o}{4\pi}\,\frac{I}{R}\,[-\cos{\varphi}]_\alpha^\beta,\] \[B= \frac{\mu_o}{4\pi}\,\frac{I}{R}\,(\cos{\alpha}-\cos{\beta}).\]

  This is the formula for the magnetic B-field of a straight conductor of finite length carrying a current at a general point P.

  We move the endpoints A and B to infinity in the case of the straight infinite conductor. This means that α = 0° and β = 180°

  \[B= \frac{\mu_o}{4\pi}\,\frac{I}{R}\,(\cos\,0^{\circ}-\cos\,180^{\circ})=\frac{\mu_o}{4\pi}\,\frac{I}{R}\,\left(1-(-1)\right).\]

  And we get the well known formula for the magnetic B-field of an infinite conductor

  \[B= \frac{\mu_o}{2\pi}\,\frac{I}{R}.\]

• Solution 2

  We can also assess the magnitude of the resulting B-field by integrating of the Biot-Savart law over other variables. We show below how to obtain the formula for the magnetic field of the infinite conductor carrying a current at point P by integrating over x.

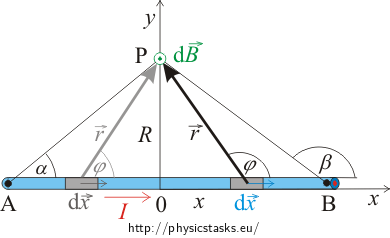
  We segment the lenght of the infinitely long conductor into an infinite number of pieces as indicated in the figure below.

  

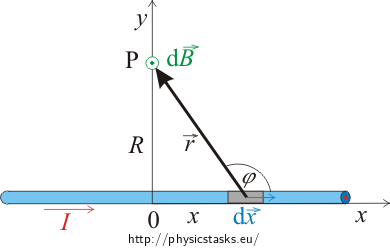
  Equation (1) that we have derived in Solution 1 for the magnitude of the magnetic B-field is valid also in this case when we consider that the ends A and B lie in infinity now. Further, it is enough to integrate over one half of the conductor this time:

  \[B=\int_{-\infty}^\infty \mathrm{d}B=2\int_0^\infty \mathrm{d}B = \frac{\mu_oI}{2\pi}\, \int_0^\infty\frac{\sin {\varphi}\, \mathrm{d}x}{r^{2}}.\tag{2}\]

  Variables r, x and angle φ are bound together in this equation by relations that are apparent from the figure. We express r and φ using x in this case.

  \[r=\sqrt{x^2+R^2},\] \[\sin{\varphi}=\frac{R}{\sqrt{x^2+R^2}}.\]

  We substitutes these relations into Equation (2)

  \[B=\frac{\mu_oI}{2\pi}\, \int_0^\infty \frac{R}{\sqrt{x^2+R^2}^{3}}\,\mathrm{d}x ,\]

  and we get by integrating:

  \[B=\frac{\mu_oI}{2\pi}\,\left[\frac{x}{R\sqrt{x^2+R^2}}\right]_0^\infty=\frac{\mu_oI}{2\pi R}.\]

  which is the formula for the magnitude of the B-field of an infinited conductor carrying a current at a general point P, which we wanted to derive.

  Note: We must pay attention to express correctly not only the magnitudes of the variables but also their signs.

• Performing the integration over x

  To simplify notation, we calculate the integral in the formula for the magnetic B-field separately, first as an idefinite integral and then over the integration interval. We label the integral J

  \[B=\frac{\mu_oI}{2\pi}\, \int_0^\infty \frac{R}{\sqrt{x^2+R^2}^{3}}\,\mathrm{d}x,\] \[J=\int \frac{R}{\sqrt{x^2+R^2}^{3}}\,\mathrm{d}x.\]

  We use the following substitution:

  \[z=1+\frac{R^2}{x^2},\] \[\mathrm{d}z=\frac{-2R^2}{x^3}\,\mathrm{d}x.\]

  We factor an x out of the square root in the denominator of J. This ajdusts the integrand into a form fitting to the proposed substution.

  \[J=\int \frac{R}{\left(x\sqrt{1+\frac{R^2}{x^2}}\right)^{3}}\,\mathrm{d}x=\int \frac{R}{x^3}\,\frac{\mathrm{d}x}{\left(\sqrt{1+\frac{R^2}{x^2}}\right)^{3}}.\]

  After substituting and integrating we get:

  \[J=\int-\frac{R}{2R^2}\,\frac{\mathrm{d}z}{\left(\sqrt{z}\right)^{3}}= \frac{1}{R}\,\frac{1}{\sqrt{z}}+c.\]

  We replace the variable z back with the substitution

  \[J=\frac{1}{R\sqrt{1+\frac{R^2}{x^2}}}+c\]

  and we rearrange by multiplicating with x/x

  \[J= \frac{x}{Rx\sqrt{1+\frac{R^2}{x^2}}}+c= \frac{x}{R\sqrt{x^2+R^2}}+c.\]

  We have got the primitive function and we find the value of the integral by using the proper integration interval

  \[J=\left[\frac{x}{R\sqrt{x^2+R^2}}+c\right]_0^\infty=\frac{1}{R}.\]

  Note: The calculation is the same for a finite conductor, only a different integration interval is used in the last step.

• Answer

  The magnitude of the magnetic B-field at any point P in the distance R from the long straight conductor of finite length carrying the current I is:

  \[B= \frac{\mu_o}{4\pi}\,\frac{I}{R}\,(\cos{\alpha}-\cos{\beta}).\]

  A special case is the conductor of infinite length with the ends moved to infinity. The magnitude of the magnetic B-field at any point P in the distance R from the infinitely long straight conductor carrying the current I is:

  \[B= \frac{\mu_o}{2\pi}\,\frac{I}{R}.\]