Perfectly Elastic Collision
Task number: 1979
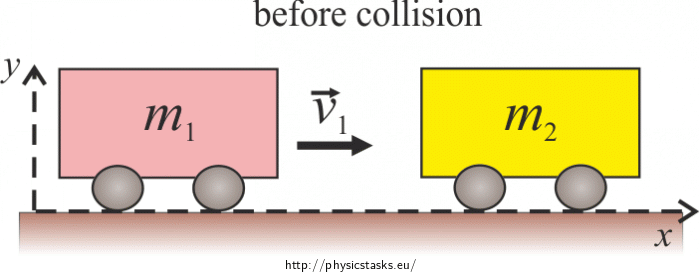
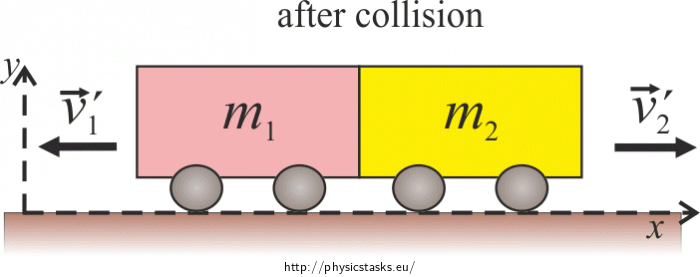
A cart on a wind trail collides elastically with another cart, which was at rest until the collision occured. After the collision both carts move at the same speed in opposite directions. Find the ratio of the masses of both carts.
Assumption
Let us assume that the carts follow a straight line:
the velocities before the collision: \[\vec{v_{\mathrm{1}}},\ \vec{v_{\mathrm{2}}}\,=\, 0\ \mathrm{m s^{-1}} \]
the velocities after the collision: \[ v_{\mathrm{1}}^{'}\, =\, v_{\mathrm{2}}^{'},\ \vec{v_{\mathrm{1}}^{'}}\, = \, -\vec{v_{\mathrm{2}}^{'}}\]
Hint 1 – Laws of conservation
Which two laws of conservation can we use to describe a perfectly elastic collision?
Hint 2 – The Law of Momentum Conservation and the Law of Mechanical Energy Conservation
Apply both laws to this scenario.
Hint 3 – Ratio of masses
From equations (1) and (2) express the ratio \[\frac{m_{\mathrm{1}}}{m_{\mathrm{2}}}.\] .
Answer
The ratio of the masses of the carts is equal to \[ \frac {m_2} {m_1} \,=\, 3\] .
Overall solution
Let us assume that the carts follow a straight line:
the velocities before the collision: \[\vec{v_{\mathrm{1}}},\ \vec{v_{\mathrm{2}}}\,=\, 0\ \mathrm{m s^{-1}} \]
the velocities after the collision: \[ v_{\mathrm{1}}^{'}\, =\, v_{\mathrm{2}}^{'},\ \vec{v_{\mathrm{1}}^{'}}\, = \, -\vec{v_{\mathrm{2}}^{'}}\]
For perfectly elastic collisions the following applies:
The Law of Momentum Conservation (LMC):
The sum of momentums of all bodies in an isolated system is constant, in other words the total momentum of an isolated system is conserved.
The Law of Mechanical Energy Conservation (LMEC):
The total mechanical energy in an isolated system is conserved, in other words the sum of potential and kinetic energy is constant.
The Law of Momentum Conservation:
\[\qquad \vec{p_\mathrm{1}} \,+\, \vec{p_\mathrm{2}}\ \,=\,\ \vec{p_\mathrm{1}^{'}} \,+\, \vec{p_\mathrm{2}^{'}} \,,\]where \(\hspace{20px} \vec{p_{\mathrm{1}}}\) – momentum of the first cart before the collision,
\(\hspace{20px} \vec{p_{\mathrm{2}}}\) – momentum of the second cart before the collision,
\(\hspace{20px} \vec{p_{\mathrm{1}}^{'}}\) – momentum of the first cart after the collision,
\(\hspace{20px} \vec{p_{\mathrm{2}}^{'}}\) – momentum of the second cart after the collision.
We substitute for both momenta:
\[m_{\mathrm{1}}\vec{v_{\mathrm{1}}}\,+\, 0\,=\,m_{\mathrm{1}}\vec{v_{\mathrm{1}}^{'}}\,+\,m_{\mathrm{2}}\vec{v_{\mathrm{1}}^{'}}\,.\]We choose a coordinate system according to the picture and rewrite the equation above using scalars
\[m_{\mathrm{1}}v_{\mathrm{1}}\,=\,\,-m_{\mathrm{1}}v_{\mathrm{1}}^{'}\,+\,m_{\mathrm{2}}v_{\mathrm{1}}^{'}\tag{1}\]The Law of Mechanical Energy Conservation:
\[ \qquad E_{\mathrm{k1}}+E_{\mathrm{k2}}=E_{\mathrm{k1}}^{'}+E_{\mathrm{k2}}^{'}\,,\]where \( \hspace{15px} E_{\mathrm{k1}}\) – kinetic energy of the first cart before the collision,
\(E_{\mathrm{k2}}\) – kinetic energy of the second cart before the collision,
\(E_{\mathrm{k1}}^{'}\) – kinetic energy of the first cart after the collision,
\(E_{\mathrm{k2}}^{'}\) – kinetic energy of the second cart after the collision.
We substitute for kinetic energy
\[\ \frac{1}{2}m_{\mathrm{1}}v_{\mathrm{1}}^{2}\,+\,0\,=\,\frac{1}{2}m_{\mathrm{1}}v_{\mathrm{1}}^{'2}\,+\,\frac{1}{2}m_{\mathrm{2}}v_{\mathrm{1}}^{'2}\,.\tag{2}\]We adjust equation (1) by factoring out \( v_{1}^{'}\) on the right side and making the square. We multiply equation (2) by two.
\[\mathrm{LMC}:\hspace{10px} m_{1}v_{1}\,=\,-m_{1}v_{1}^{'}\,+\,m_{2}v_{1}^{'}\,=\,v_{1}^{'}(m_{2}-m_{1}) \hspace{25px}\,/^2\] \[\mathrm{LMEC}:\hspace{10px} \frac{1}{2}m_{1}v_{1}^{2}\,=\,\frac{1}{2}m_{1}v_{1}^{'2}\,+\,\frac{1}{2}m_{2}v_{1}^{'2} \hspace{25px}\,/\cdot 2\]We now have the equations in a form:
\[\ m_{1}^{2}v_{1}^{2}\,=\,v_{1}^{'2}(m_{2}-m_{1})^{2}\] \[ m_{1}v_{1}^{2}\,=\,v_{1}^{'2}(m_{1}\,+\,m_{2})\]We evaluate \(v_{1}^{2}\) from both equations and put them equal to each other.
\[\frac{v_{1}^{'2}}{m_{1}^{2}}(m_{2}-m_{1})^{2}\,=\,\frac{v_{1}^{'2}}{m_{1}}(m_{1}\,+\,m_{2})\]Because \(\frac{v_{1}^{'2}}{m_{1}^{2}}\) is never zero, we can divide the equation by it.
\[(m_{2}-m_{1})^{2}\,=\,m_{1}(m_{1}\,+\,m_{2})\]All we have to do now is rearrange the equation:
\[m_{2}^{2}-2m_{1}m_{2}\,+\,m_{1}^{2}\,=\,m_{1}^{2}\,+\,m_{1}m_{2}\] \[m_{2}^{2}-3m_{2}m_{1}\,=\,0\] \[m_{2}(m_{2}-3m_{1})\,=\,0\]Because the mass of the second cart is never zero, it must hold that:
\[m_{2}\,=\,3m_{1}\,.\]The ratio of the masses is then equal to:
\[\frac{m_{2}}{m_{1}}\,=\,3\,.\]Answer: The mass ratio of the carts is \( \frac{m_{2}}{m_{1}}\,=\,3\,.\)