The Voyage of a Raft
Task number: 2000
A raft is sailing across the river perpendicularly to its current. It is moving with a constant speed w relative to water. The width of the river is d. Assume that water is drifting the raft so the component of the velocity of the raft caused by the flowing water grows quadratically with the distance from the riverbanks. It is equal to zero at the riverbank and it reaches its maximum value u in the middle of the river.
Determine the time dependence of the velocity \(\vec{v}(t)\) of the raft relative to the riverbank and the dependence of the raft’s position \(\vec{r}(t)\) on time.
Where exactly on the opposite bank is the raft going to land?
Hint 1: A picture of the situation
Draw a picture of the situation. Mark coordinate axes in it and split the motion of the raft into motion in the direction of x axis (perpendicular to the current) and into motion in the direction of y axis (as the river flows).
Draw the behaviour of the component of the raft velocity vy caused by the flowing water.
Hint 2: Behaviour of the velocity of the raft
Determine the components of the velocity vx of the raft and vy, using the picture in the hint above.
Which function represents the velocity in the direction of the y axis?
Hint 3: Time behaviour of the position of the raft
You know the components of the raft velocity. Determine the coordinates of the raft and the time behaviour of its position vector from them.
Hint 4: A location of a place where the raft is going to land
You need to find the coordinates of the place where the raft is going to land on the other riverbank. You know the width of the river so you know also the x coordinate. The components of the velocity of the raft are also known to you. Express the time which it takes the raft to cross the river. Then you can also determine the y coordinate at this time.
Complete solution
Picture 1 – the situation:
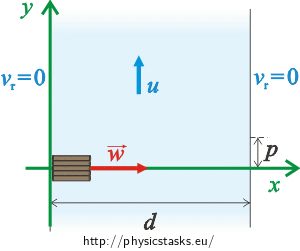
Picture 2 – the behaviour of y component of the raft velocity caused by the flowing water:

In the picture 1, vr represents the speed of river current, the speed of the raft in respect to riverbanks is v, the components of the velocity of the raft are vx, vy.
It is a superposition of two movements (in the direction of x and y axes).
Behaviour of the velocity of the raft
According to pictures 1, 2 it holds:
\[v_x\,=\,w,\tag{1}\] \[v_y\,=\,ax^{2}\,+\,bx\,+\,c.\tag{2}\]The raft velocity in the direction of y axis as a function of the distance from the first bank forms a parabolic curve.
Determining the coefficients a, b, c:
We know some points on the parabola: \(\left[0,\,0\right]\), \(\left[\frac{d}{2},\,u\right]\), \(\left[d,\,0\right]\).
Substituting the point [0, 0] we get:
\[c\,=\,0\,.\]Substituting the point \(\left[\frac{d}{2},\,u\right]\) we get:
\[a\left(\frac{d}{2}\right)^{2}\,+\,b\frac{d}{2}\,=\,u\,.\tag{3}\]Substituting the point [d, 0] we get:
\[ad^{2}\,+\,bd\,=\,0\,.\tag{4}\]We get a system of two equations with two variables a,b:
From (4):
\[a\,=\, -\frac{b}{d}\,.\]We substitute in (3):
\[-\frac{bd^{2}}{4d} \,+\, \frac{bd}{2} \,=\, u,\] \[\frac{bd}{4} \,=\, u,\] \[b\,=\,\frac{4u}{d},\] \[a\,=\,-\frac{4u}{d^{2}}.\]We substitute the coefficients in (2):
\[v_y \,=\, -\frac{4u}{d^{2}}x^{2}\,+\,\frac{4u}{d}x\,,\]We know from (1) that \(x\,=\,wt\,.\) Thus:
\[v_y\,=\,-\frac{4u}{d^{2}}w^{2}t^{2}\,+\,\frac{4u}{d}wt,\tag{5}\]
\[\vec{v}(t)\,=\,v_x\vec{i} \,+\, v_y\vec{j},\] \[\vec{v}(t)\,=\,w\vec{i}\,+\,\left(\frac{4u}{d}wt\,-\,\frac{4u}{d^{2}}w^{2}t^{2}\right)\vec{j},\]Where \(\vec{i}\), \(\vec{j}\) are the unit vectors in the direction of the coordinate axes.
Time behaviour of the position of the raft
The components of the velocity of the raft according to (1) and (5) are:
\[v_x\,=\,w,\] \[v_y\,=\,-\frac{4u}{d^{2}}\,w^{2}t^{2}\,+\,\frac{4u}{d}\,wt.\]Then the coordinates are:
\[x\,=\, wt,\] \[y\,=\,\int{v_y}\,\mathrm{d}t\,=\,\int\left(-\frac{4u}{d^{2}}w^{2}t^{2}\,+\,\frac{4u}{d}wt\right)\,\mathrm{d}t\,=\, -\frac{4u}{3d^{2}}w^{2}t^{3}\,+\,\frac{4u}{2d}wt^{2}\,+\,k.\]For t = 0 s is y = 0 m, thus k = 0:
\[y \,=\, -\,\frac{4u}{3d^{2}}\,w^{2}t^{3}\,+\,\frac{2u}{d}\,wt^{2},\tag{6}\]
\[\vec{r}(t)\,=\,x\vec{i}\,+\,y\vec{j}\,,\] \[\vec{r}(t)\,=\,wt\vec{i}\,+\,\left(\frac{2u}{d}\,wt^{2}\,-\,\frac{4u}{ 3d^{2}}\,w^{2}t^{3}\right)\vec{j}\,,\]where \(\vec{i}\), \(\vec{j}\) are the unit vectors in the direction of the coordinate axes.
A location of a place where the raft is going to land
The raft gets across the river in the time \(t \,=\, \frac{d}{w}\) since it is supposed to cover the distance d moving at velocity w in the direction of the x axis.
Coordinates of the place where the raft is going to land are:
\[x\,=\,d\,.\]The y coordinate at time \(t \,=\, \frac{d}{w}\) is according to (6):
\[y \,=\, \frac{2uw}{d}t^{2}\,-\,\frac{4uw^{2}}{3d^{2}}t^{3} \,=\, \frac{2uw}{d}\,\frac{d^{2}}{w^{2}} \,-\,\frac{4uw^{2}}{3d^{2}}\,\frac{d^{3}}{w^{3}},\] \[y \,=\, \frac{2ud}{w} \,-\, \frac{4ud}{3w} \,=\, \frac{2ud}{3w}.\]The coordinates of the place where the raft is going to land are:
\[x\,=\,d\,,\] \[y\,=\,\frac{2ud}{3w}\,.\]Answer
Time dependence of the velocity \(\vec{v}(t)\) of the raft relative to the riverbank is:
\[\vec{v}(t)\,=\,w\vec{i}\,+\,\left(\frac{4u}{d}wt\,-\,\frac{4u}{d^{2}}w^{2}t^{2}\right)\vec{j}\,,\]where \(\vec{i}\), \(\vec{j}\) are the unit vectors in the direction of the coordinate axes.
Time behaviour of the position of the raft \(\vec{r}(t)\) is:
\[\vec{r}(t)\,=\,wt\vec{i}\,+\,\left(\frac{2u}{d}wt^{2}\,-\,\frac{4u}{ 3d^{2}}w^{2}t^{3}\right)\vec{j}\,,\]where \(\vec{i}\), \(\vec{j}\) are the unit vectors in the direction of the coordinate axes.
The position of the place where the raft is going to land at the other riverbank is:
\[x\,=\,d\,,\] \[y\,=\,\frac{2ud}{3w}\,.\]




