Collection of Solved Problems in Physics

Decomposition of Forces

Task number: 2229

Weight F_G is acting on a body attached to two rods K and L (see Fig. 1).

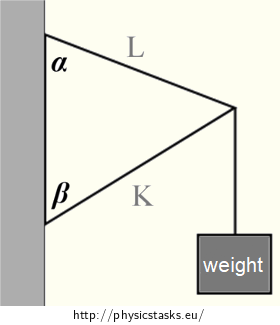
Figure 1

Express the magnitudes of forces exerted on the rods K and L.

• Hint 1

  Which forces act at the contact point of the rods and suspension cord and in which direction? Draw them in the picture. What is the resultant force?

• Solution of Hint 1

  The following forces are present at the contact point of the rods and suspension cord (see Fig. 2):

  • pushing force K in the direction of rod K,
  • pulling force L in the direction of rod L,
  • weight of the body in the vertical direction G = F_G.

  

  Since the suspension cord is at rest with respect to the supporting structure, the resultant force must equal to zero:

  \[\vec{F}_G\,+\,\vec{K}\,+\,\vec{L}\,=\,\vec{o}.\]

• Hint 2

  Draw the resultant of forces K and L in Figure 2. Its magnitude is known. Mark angles α and β in the arisen parallelogram of forces. Select one of the triangles in which you know the magnitude of all three angles and one side. Can we apply some mathematical rule to this triangle (which is generally not rectangular) and express the length of two remaining sides which correspond to the magnitudes of forces K and L?

• Solution of Hint 2

  Forces F_G, K and L form triangle ABC, shown in Figure 3. Since we know the magnitude of all its angles and side AB of the triangle, we can apply the sine rule to it.

  

• Hint 3

  Write the sine rule for triangle ABC and express the magnitude of forces K and L from it.

• Solution of Hint 3

  Using the sine law for triangle ABC, we get:

  \[\frac{K}{\sin{\alpha}}\,=\,\frac{L}{\sin{\beta}}\,=\,\frac{F_G}{\sin({\pi\,-\,(\alpha\,+\,\beta))}}.\tag{1}\]

  Sine function has the following property:

  \[\sin{(\pi\,-\,(\alpha\,+\,\beta))}\,=\,\sin(\alpha\,+\,\beta).\tag{2}\]

  Thus, combining equations (1) and (2), we obtain:

  \[K\,=\,\frac{F_G\sin{\alpha}}{\sin(\alpha\,+\,\beta)},\] \[L\,=\,\frac{F_G\sin{\beta}}{\sin(\alpha\,+\,\beta)}.\]

• Overall Solution

  The following forces are present at the contact point of the rods and suspension cord (see Fig. 2):

  • pushing force K in the direction of rod K,
  • pulling force L in the direction of rod L,
  • weight of the body in the vertical direction G = F_G.

  

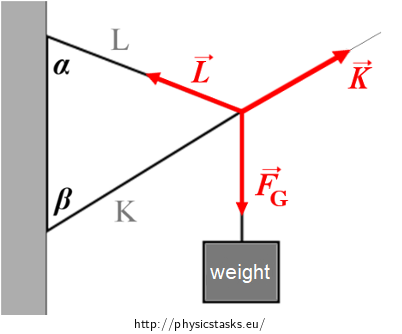
  Since the suspension cord is at rest with respect to the supporting structure, the resultant force must equal to zero:

  \[\vec{F}_G\,+\,\vec{K}\,+\,\vec{L}\,=\,\vec{o}.\]

  Forces F_G, K and L form the triangle ABC, shown in Figure 3.

  

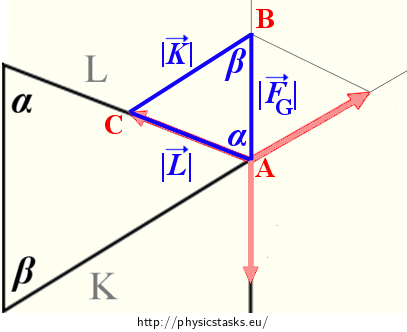
  Since we know the magnitude of all its angles and side AB of the triangle, we can apply the sine rule on it:

  \[\frac{K}{\sin{\alpha}}\,=\,\frac{L}{\sin{\beta}}\,=\,\frac{F_G}{\sin({\pi\,-\,(\alpha\,+\,\beta))}}.\tag{1}\]

  Sine function has the following property:

  \[\sin{(\pi\,-\,(\alpha\,+\,\beta))}\,=\,\sin(\alpha\,+\,\beta).\tag{2}\]

  Thus, combining relations (1) and (2), we obtain:

  \[K\,=\,\frac{F_G\sin{\alpha}}{\sin(\alpha\,+\,\beta)},\] \[L\,=\,\frac{F_G\sin{\beta}}{\sin(\alpha\,+\,\beta)}.\]

• Answer

  Magnitude of forces K and L can be expressed as follows:

  \[K\,=\,\frac{F_G\sin{\alpha}}{\sin(\alpha\,+\,\beta)},\] \[L\,=\,\frac{F_G\sin{\beta}}{\sin(\alpha\,+\,\beta)}.\]