Ice Cube and Time Varying Force
Task number: 1980
An ice cube with a mass of 10 kg is acted upon by a horizontal force dependent on time. This dependence is described with the formula Fx = p(q − t) , where p = 100 N·s−1, q = 1 s. At time t = 0 s the ice cube was at the beginning of our frame of reference, its speed was 0.2 m·s−1 and the force was aligned with its velocity. The ice cube is moving on a horizontal ice plane with no friction.
Note: Hereafter, acceleration refers to the magnitude of acceleration and speed the magnitude of velocity.
Given values
m = 10 kg mass of the ice cube Fx = p(q − t) force acting upon the cube p = 100 N·s−1 constant q = 1 s constant v0 = 0,2 m·s−1 initial speed of the cube tx = ? duration of the movement of the cube xx = ? distance travelled by the cube Hint 1 – Acceleration of the ice cube
Which forces act upon the ice cube? Write down the equation of motion and evaluate the acceleration.
Hint 2 – Dependence of speed and distance on time
We obtained the acceleration in relation (2). Speed of the ice cube can be obtained by integrating acceleration, and distance by integrating speed.
Hint 3 – The time of stopping
What is the speed of the ice cube at the moment it stops moving?
Hint 4 – The distance travelled by the cube
We know the time of stopping and the dependence of traveled distance on time. We calculate the distance of stopping by substituting the time of stopping into this relation.
OVERALL SOLUTION
We draw the forces acting upon the ice cube into a picture and write the equation of motion for the cube.
Forces acting upon the ice cube:
\(\vec{F}_{\mathrm{G}}\)…gravitational force
\(\vec{N}\)…force by which the plane acts upon the ice cube
\(\vec{F}_{\mathrm{x}}\)…time varying force acting upon the ice cube
Equation of motion for the ice cube:
\[\vec{F}_{\mathrm{x}}+\vec{F}_{\mathrm{G}}+\vec{N}\,=\, m\vec{a}\tag{1}\]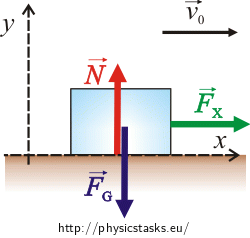
We rewrite the equation using scalars. We choose the x axis in the direction of the motion of the ice cube. The y axis is perpendicular to the x axis:
\[x:\qquad F_\mathrm{x} \,=\, ma\] \[(q-t) \,=\, ma\] \[\frac{p(q-t)}{m} \,=\, a\tag{2}\] \[y:\qquad N - F_\mathrm{G}\,=\,0\tag{3}\](the acceleration of the cube in the direction of the y axis is zero)
We obtain the dependence of speed on time by integrating the acceleration from relation (2):
\[v(t)=\int{a(t)}\,dt\] \[v(t)\,= \int{\frac{p(q-t)}{m}}\,\mathrm{d}t\,= \int{\frac{pq}{m}}\,\mathrm{d}t\,-\int{\frac{pt}{m}}\,\mathrm{d}t\,= \frac{pgt}{m}\,-\,\frac{pt^2}{2m}+C\tag{4}\]At time t = 0 s the speed is equal to vo. It therefore holds that:
\[v_\mathrm{0}\,=\,\frac{pg}{m}\cdot0\,-\,\frac{p\cdot0}{2m}\,+\,C\]From here:
\[v_\mathrm{0}\,=\,C\]By substituting to relation (4) we obtain:
\[v(t)\,=\,\frac{pgt}{m}\,-\,\frac{pt^2}{2m}\,+\,v_0\tag{5}\]We get the dependence of distance on time by integrating the speed from relation (5):
\[x(t)\,=\,\int{v(t)}\,\mathrm{d}t\] \[x(t)\,=\, \int{(\frac{pqt}{m}\,-\,\frac{pt^2}{2m}\,+\,v_\mathrm{0}})\,\mathrm{d}t\,=\, \frac{pqt^2}{2m}\,-\,\frac{pt^3}{6m}\,+\,v_\mathrm{0}t\,+\,K\tag{6}\]At time t = 0 s the distance is 0 m. It therefore holds that:
\[0\,=\,\frac{pq\cdot0}{2m}\,-\,\frac{p\cdot0}{6m}\,+\,v_\mathrm{0}\cdot0\,+\,K\]From here:
\[0\,=\,K\]By substituting into relation (6) we get:
\[x(t)\,=\,\frac{pqt^2}{2m}\,-\,\frac{pt^3}{6m}\,+\,v_\mathrm{0}t\tag{7}\]At the time the cube stops, its speed is zero.
Due to relation (5), for the time txthe ice cube stops it holds that:
\[0=\frac{pqt_\mathrm{x}}{m}-\frac{pt_z^2}{2m}+v_\mathrm{0}\]We obtain a quadratic equation with the unknown tx:
\[0=-\frac{pt_\mathrm{x}^2}{2m}+\frac{pqt_\mathrm{x}}{m}+v_\mathrm{0}\tag{8}\]We solve the equation (8). First, we calculate the discriminant:
\[D=\frac{p^2q^2}{m^2}-4\left(-\frac{v_\mathrm{0}p}{2m}\right)= \frac{p}{m}\left(\frac{pq^2}{m}+2v_\mathrm{0}\right)\]Then we calculate the unknown tx. We obtain 2 mathematical solutions:
\[t_{\mathrm{x_1}}=\frac{-\frac{pq}{m}+\sqrt{D}}{-\frac{p}{m}}\] \[t_{\mathrm{x_2}}=\frac{-\frac{pq}{m}-\sqrt{D}}{-\frac{p}{m}}\]By substituting for D we get:
\[t_{\mathrm{x_1}}=\frac{-\frac{pq}{m}+\sqrt{\frac{p}{m} \left(\frac{pq^2}{m}+2v_\mathrm{0}\right)}}{-\frac{p}{m}}=q-\frac{m}{p}\sqrt{\frac{p}{m} \left(\frac{pq^2}{m}+2v_\mathrm{0}\right)}\] \[t_{\mathrm{x_1}}=q-\sqrt{q^2+\frac{2mv_\mathrm{0}}{p}}\tag{9}\] \[t_{\mathrm{x_2}}=\frac{-\frac{pq}{m}-\sqrt{\frac{p}{m}\left(\frac{pq^2}{m}+2v_\mathrm{0}\right)}}{-\frac{p}{m}}= q+\frac{m}{p}\sqrt{\frac{p}{m}\left(\frac{pq^2}{m}+2v_\mathrm{0}\right)}\] \[t_{\mathrm{x_2}}=q+\sqrt{q^2+\frac{2mv_\mathrm{0}}{p}}\tag{10}\]We substitute numerically to relations (9) and (10):
\[t_{\mathrm{x_1}}=1-\sqrt{1^2+\frac{2{\cdot}10\cdot0.2}{100}}=1-\sqrt{\frac{104}{100}}= 1-1.02=-0.02\,\textrm{s}\] \[t_{\mathrm{x_2}}=1+\sqrt{1^2+\frac{2{\cdot}10\cdot0.2}{100}}=1+\sqrt{\frac{104}{100}}= 1+1.02=2.02\,\textrm{s}\tag{11}\]Only the positive time \(t_{\mathrm{x_2}}\) from the result (11) bears any physical significance.
We know the time the ice cube stops moving tx2 = tx from result (11) and the dependence of traveled distance on time from relation (7):
\[x(t)=\frac{pqt^2}{2m}\,-\,\frac{pt^3}{6m}+v_\mathrm{0}t\tag{7}\] \[t_\mathrm{x}=2.02\,\mathrm{s}\tag{11}\]We calculate the distance the ice cube travelled by substituting the time of stopping (11) into relation (7):
\[x(t_\mathrm{x})=\frac{pqt_\mathrm{x}^2}{2m}\,-\,\frac{pt_\mathrm{x}^3}{6m}+v_\mathrm{0}t_\mathrm{x}\]We substitute numerically:
\[x(t_\mathrm{x})=\left(\frac{100{\cdot}1\cdot(2.02)^2}{2{\cdot}10}\,-\, \frac{100\cdot\left(2.02\right)^3}{6{\cdot}10}\,+\,0.2{\cdot}2.02\right)\,\textrm{m}\] \[x(t_\mathrm{x})=\left(20.40\,-\,13.74\,+\,0.40\right)\,\textrm{m}=7.06\,\textrm{m}\tag{12}\]Answer
The ice cube stopped in 2.02 s, 7.06 m from the beginning of our frame of reference.



