Collection of Solved Problems in Physics

Passing of a train I

Task number: 386

A passenger train of length \(L=120\,\mathrm{m}\) travels at a speed of \(v=30\,\mathrm{\frac{km}{h}}\). How long does it take the train to pass:

a)  a power pole?

b)  a platform of length \(d=50\,\mathrm{m}\)?

• Hint 1:

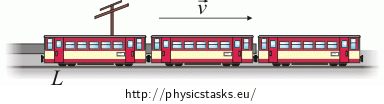
  Think of what distance the locomotive of the train has to travel so that the whole train passes the pole or the platform.

  Draw a picture for both cases.

• Solution of Hint 1:

  a)   We suppose that the width of the pole is small compared with the length of the train. The locomotive has to travel the whole length of the train to pass the pole.

  

  b)   The locomotive has to travel the length of the whole train plus the length of the platform in order to the whole train passes it.

  

• Hint 2:

  You know the distance which the locomotive has to travel and its speed. The desired times can be found easily.

  Make sure that all the assigned quantities are in the base units.

• Solution of Hint 2:

  Convert the speed of the locomotive to the base units:

  \[ v\,=\,30\,\mathrm{\frac{km}{h}}\,\dot=\, 8.3\,\mathrm{\frac{m}{s}}\,. \]

  The other assigned values are in the base units.

  Determine the desired times by dividing the distance which the locomotive has to travel by the speed of the train:

  a) \(t_{1}\,=\,\frac{L}{v}\,=\,\frac{120}{8.3}\,\mathrm{s}\,=\,14.4\,\mathrm{s}.\)

  b) \( t_{2}\,=\,\frac{L+d}{v}\,=\,\frac{120+50}{8.3}\,\mathrm{s}\,=\,20.4\,\mathrm{s} \)

• Complete Solution a)

  a) Suppose that the width of the pole is very small compared with the length of the train. Before the whole train passes the pole, the locomotive has to travel the distance equal to the length of the train, i.e. L.

  

  It travels this distance in the time:

  \[t_{1}\,=\,\frac{L}{v}\,=\,\frac{120}{8{,}3}\,\mathrm{s}\,=\,14.4\,\mathrm{s}.\]

• Complete Solution b)

  b) We cannot consider the width of the platform insignificant, we have to take it into account. In order for the whole train pass the platform, the locomotive has to travel the length of the train L (so the whole train passes the beginning of the platform) and the length of the platform d (the whole train passes the whole platform).

  

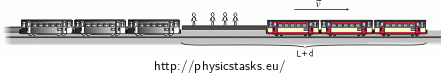
  It travels this distance in the time:

  \[ t_{2}\,=\,\frac{L+d}{v}\,=\,\frac{120+50}{8.3}\,\mathrm{s}\,=\,20.4\,\mathrm{s} \]

• Complete answer

  The passenger train passes the power pole in \(14.4\,\mathrm{s}\). The train passes the platform in \(20.4\,\mathrm{s}\).

• Reference to a similar task

  A similar but slightly more difficult task about the passing of two moving trains is Passing of a train II.